Question
A bullet in a gun is accelerated from the firing chamber to the end of the barrel at an average rate of for . What is its muzzle velocity (that is, its final velocity)?
Final Answer
Solution video
OpenStax College Physics, Chapter 2, Problem 22 (Problems & Exercises)
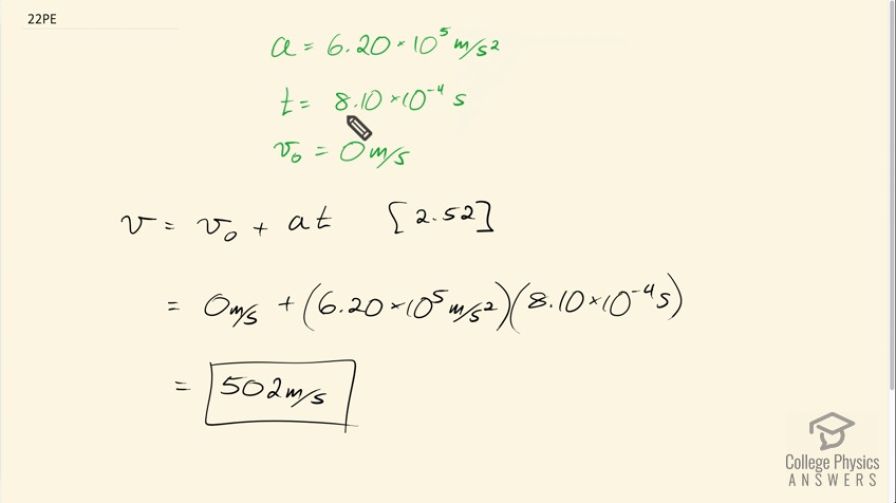
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The bullet in this gun is accelerating at 6.20 times 10 to the 5 meters per second squared and for a time of 8.10 times 10 to the minus 4 seconds; we assume that the initial velocity of the bullet must be zero because it's starting at rest inside the gun. Now its final velocity then will be the initial velocity plus acceleration times time—that's equation 52 from chapter 2— and that is 0 meters per second plus 6.20 times 10 to the 5 meters per second squared times 8.10 times 10 to the minus 4 seconds of time giving a final velocity of 502 meters per second which is very fast; it is greater than the speed of sound which is 340 meters per second on a typical day.
