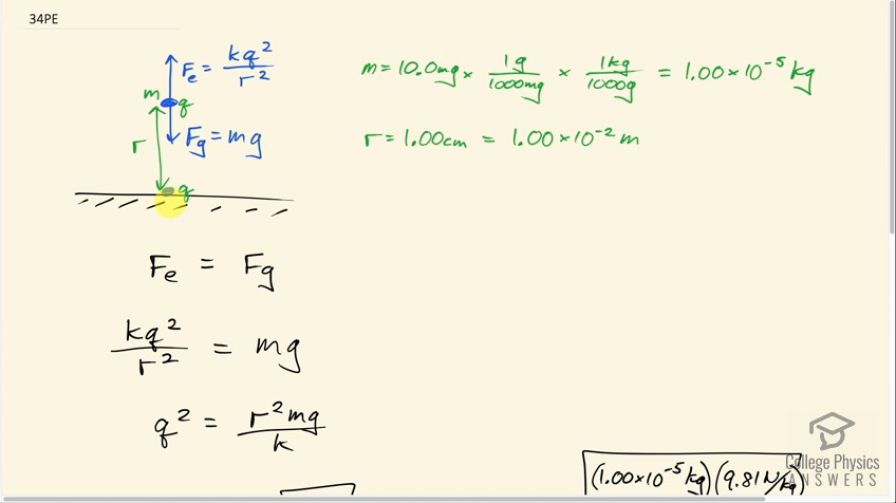
Question
(a) Common transparent tape becomes charged when pulled from a dispenser. If one piece is placed above another, the repulsive force can be great enough to support the top piece's weight. Assuming equal point charges (only an approximation), calculate the magnitude of the charge if electrostatic force is great enough to support the weight of a 10.0 mg piece of tape held 1.00 cm above another. (b) Discuss whether the magnitude of this charge is consistent with what is typical of static electricity.
Final Answer
- Question #14 (or Questions #28 if you're using the AP edition of the textbook) states that a typical static charge is 75.0 nC. The answer in part (a) is consistent with a typical static charge since it's within one order of magnitude.
Solution video
OpenStax College Physics for AP® Courses, Chapter 18, Problem 34 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Two pieces of transparent tape end up having the same charge after they are pulled from the dispenser and one piece of tape can be suspended above the other because since the charges are the same, there's going to be a force of repulsion upwards on this top charge; the bottom charge we assume is resting on the ground. and it's not gonna move anywhere because it will have a normal force upwards on it but that's you know beside the point. The point is that this top charge is suspended there which is to say that the net force on it is zero so the electrostatic repulsion upwards is going to equal the force of gravity downwards. So the force of gravity is the mass of this piece of tape times gravitational field strength and the electrostatic repulsion will be Coulomb's constant times the product of the two charges divided by the distance between them squared. So the distance between them is 1.00 centimeter which is 1.00 times 10 to the minus 2 meters and the mass of the top charge is 10 milligrams which is converted into grams by dividing by a 1000 or multiplying by 1 gram for every 1000 milligrams and then convert from grams into kilograms and this works out to 1.00 times 10 to the minus 5 kilograms. We always want mks units in our formulas— meters, kilograms and seconds. Okay! So the electrostatic repulsion upwards equals the force of gravity downwards so that means kq squared over r squared equals mg and then we can solve for q by first solving for q squared by multiplying both sides by r squared over k and then we have this line here and then take the square root of both sides to solve for q. So q is r times square root of mg over k. So that's 1.00 times 10 to the minus 2 meters times square root of 1.00 times 10 to the minus 5 kilograms times 9.81 newtons per kilograms divided by Coulomb's constant and we end up with 1.04 nanocoulombs. Now question number 14—or number 28 if you are using the A.P. edition of the OpenStacks College Physics textbook— says that a typical static charge is 75.0 nanocoulombs. So yes, one nanocoulomb is consistent with a typical static charge.
