Question
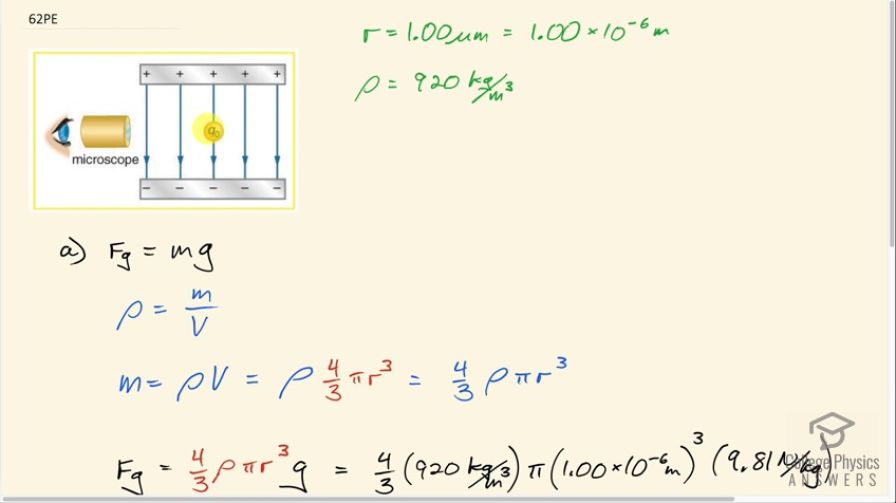
The classic Millikan oil drop experiment was the first to obtain an accurate measurement of the charge on an electron. In it, oil drops were suspended against the gravitational force by a vertical electric field. (See Figure 18.58.) Given the oil drop to be in radius and have a density of : (a) Find the weight of the drop. (b) If the drop has a single excess electron, find the electric field strength needed to balance its weight.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 18, Problem 62 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
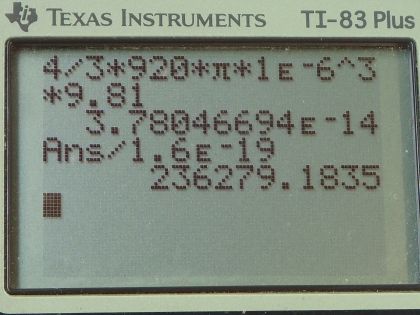
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The Millikan Oil Drop experiment was used to figure out what the charge is on a single electron but in this question, we are going to presume that we know the charge on a single electron and figure out what this electric field strength is. So when Millikan originally did this experiment, he knew what the electric field was and what the size of the oil drop was and therefore its weight and he fused those pieces of information to figure out the charge. Okay! So the radius of this drop of oil is 1.00 micrometer which is 1.00 times 10 to the minus 6 meters and the density of this oil is 920 kilograms per cubic meter. So question (a) asks us to figure out what is the weight on this drop of oil— that's going to be its mass times gravitational field strength— and we'll figure out the mass using this density formula— density is mass per volume— and we can solve for m by multiplying both sides by V. So mass is the density times the volume and then we'll use the radius to figure out what the volume is knowing this formula for the volume of a sphere— four-thirds π times its radius cubed— so this is what we'll substitute in for m in our formula for the weight. So that's four-thirds ρ times πr cubed times g. So that's four-thirds times 920 kilograms per meter cubic meter times π times 1.00 micrometer cubed times 9.81 newtons per kilogram giving a force of gravity, or weight in other words, of 3.78 times 10 to the minus 14 newtons. In part (b) it says that if the drop has a single excess electron, find the electric field strength. So the electrostatic force will be the charge times the electric field strength and that equals the force of gravity since the drop is suspended and not accelerating. So we divide both sides by q to solve for E and it's the force of gravity divided by the charge. So that's 3.7805 times 10 to the minus 14 newtons— that we found in part (a)— and divide by the charge on a single electron which is 1.60 times 10 to the minus 19 coulombs. This gives an electric field strength of 2.36 times 10 to the 5 newtons per coulomb.