Question
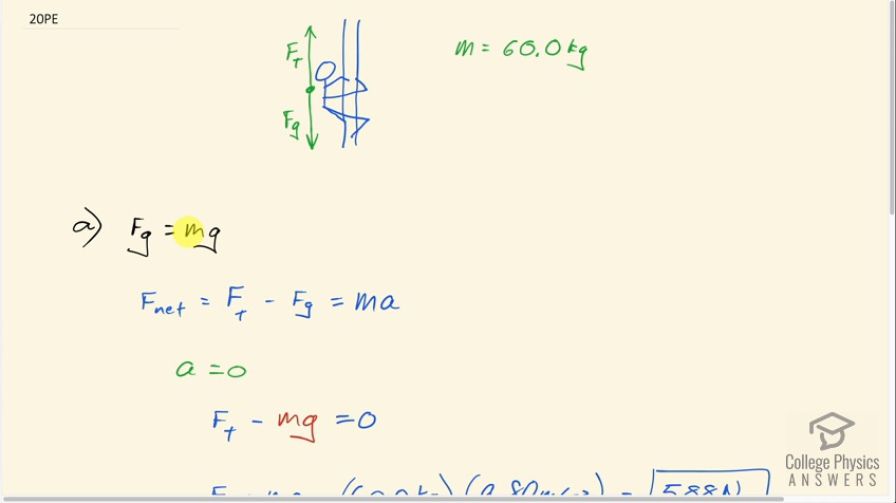
Suppose a 60.0-kg gymnast climbs a rope. (a) What is the tension in the rope if he climbs at a constant speed? (b) What is the tension in the rope if he accelerates upward at a rate of ?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 4, Problem 20 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This climber up a rope experiences two forces vertically. One is the gravity force downwards, which is their mass times acceleration due to gravity and also the force upwards due to the rope. So the tension in the rope is going to be the force upwards on the person. The net force in part A, we have acceleration is zero by the way, the net force is tension upwards minus gravity downwards. And so that's going to be mass times acceleration. But given that acceleration is zero, this term is zero on the right hand side, and we replace Fg with mg here as well. Then solve for by adding mg to both sides. And we get the tension that is the mass of 60 kilograms times acceleration due to gravity of 9.8 meters per second squared, which is a force of 588 newtons. In part B, where it said, suppose that the climber accelerates upwards at a rate of 1.5 meters per second squared. So upwards means this number is positive. We're going to substitute in positive 1.5 in place of this letter A here. So I've written down the net force in Newton's second law equation again, but I've replaced force of gravity with mg right away, and put a minus in front of it because it's directed downwards. Now we're going to add that mg to both sides to solve for the tension force. Tension force is m times g plus a, and where we factored out the m, since it's the common factor among these two terms. We replace m with 60 kilograms, and then g is 9.8 meters per second squared, and the acceleration is 1.5 meters per second squared, which gives a tension force of 678 newtons. Now as a reality check, we should confirm that this number is greater than the answer for part A, because when the person is accelerating upwards, that means there must be more force upwards applied on them than the case where their speed is constant. So we expected an answer greater than 588, and sure enough it is. So that gives us confidence that this is the correct answer.
