Question
When landing after a spectacular somersault, a 40.0-kg gymnast decelerates by pushing straight down on the mat. Calculate the force she must exert if her deceleration is 7.00 times the acceleration due to gravity. Explicitly show how you follow the steps in the Problem-Solving Strategy for Newton’s laws of motion.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 4, Problem 26 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
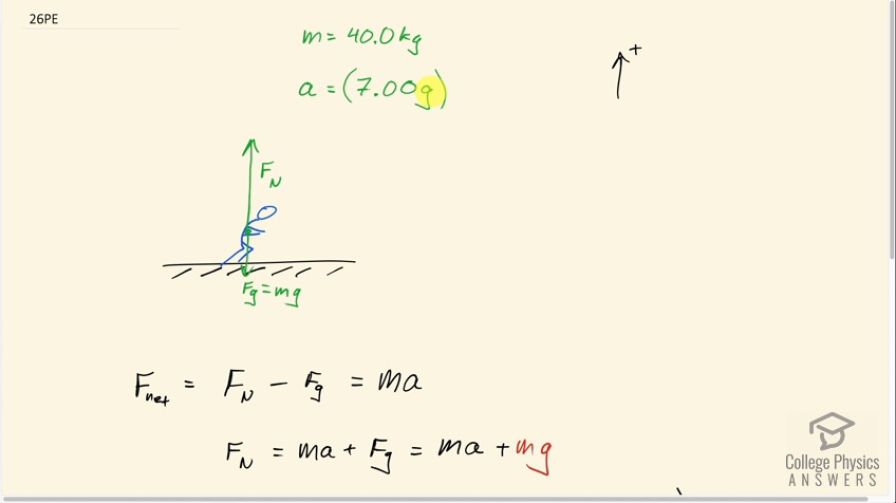
This is College Physics Answers with Shaun Dychko. A gymnast lands on the ground and experiences an acceleration upwards of magnitude seven g’s. So acceleration of 7.00 times g. The mass of the gymnast is 40 kilograms and our question is to figure out the force that they apply on the ground. Which, in the ground and then in turn applies that same force on the gymnast, but in the opposite direction upwards. So this is the normal force applied by the ground on the gymnast, and then there’s also a force of gravity downwards on the gymnast. So this is a free body diagram of the gymnast. There are two forces; one applied upwards by the ground, and the other one applied downwards by gravity. So the net force is going to be their mass times their acceleration. And this is the acceleration we’re given, and we’re also given the mass. And we can figure out what the force of gravity is, because that's their mass times gravitational field strength g. And then we’ll figure out what this normal force is. And so we’ll add f g to both sides to solve for f n. So f n and is m a plus f g and then replacing f g with mass times gravitational field strength. And then factoring out the common factor, m. We get that the normal force is mass times the sum of the acceleration plus acceleration due to gravity. I'm using the terms acceleration due to gravity and gravitational field strength sort of interchangeably. They're both represent the same thing. If you talk about gravitational field strength, then your units are newtons per kilogram is probably what you thinking of. And if you talk about acceleration due to gravity, then the units will be meters per second squared, but these are equivalent. Okay, but anyway I digress. Lets plug-in some numbers here; We have 40 kilograms times seven g, which is acceleration, plus an additional g. So seven g plus g is eight g. So we have 40 times eight, times 9.8 meters per second squared. And I guess I could write that down more explicitly; 40 kilograms times 8.00, times 9.80 meters per seconds squared; giving us a force of 3.14 times ten to the three newtons.
