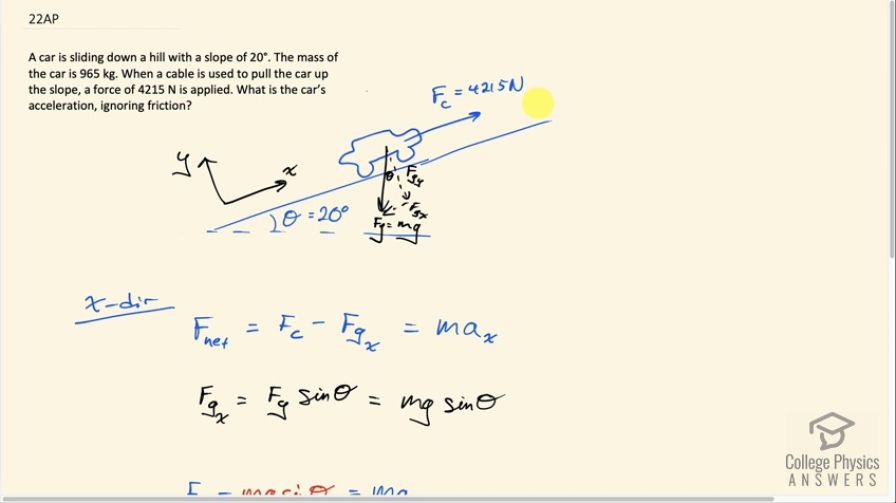
A car is sliding down a hill with a slope of $20^{\circ }$. The mass of the car is 965 kg. When a cable is used to pull the car up the slope, a force of 4215 N is applied. What is the car’s acceleration, ignoring friction?

Video Transcript

This is College Physics Answers with Shaun Dychko. A car is being pulled by a cable with the force of 4,215 newtons up the slope, and there's also going to be some component of gravity, which is directed down the slope. The slope's inclined at 20 degrees. The car is a massive 965 kilograms. We're going to define our axis so that the x-axis is along the slope. When we talk about F gx, we're talking about the component of gravity along the slope in other words, the parallel component of gravity. The net force in the x-direction equals the cable force upwards minus the force due to gravity backwards, and that equals mass of the car times its acceleration in the x-direction. This x-component is the force of gravity F g, which is the hypotenuse of this right triangle. We're going to have to multiply by sine of this angle theta to get this opposite leg right here. That is mg is what gravity is times sine theta and then replace that into F gx in this net force, Newton's second law formula. We do that here. Then we divide both sides by m to solve for the acceleration and switch the sides around as well. The acceleration is the cable force minus mg sine theta over m. It's 4,215 newtons minus 965 kilograms times 9.8 meters per second squared times sine 20 degrees divided by the mass, which is 1.02 meters per second squared, up the slope.