Question
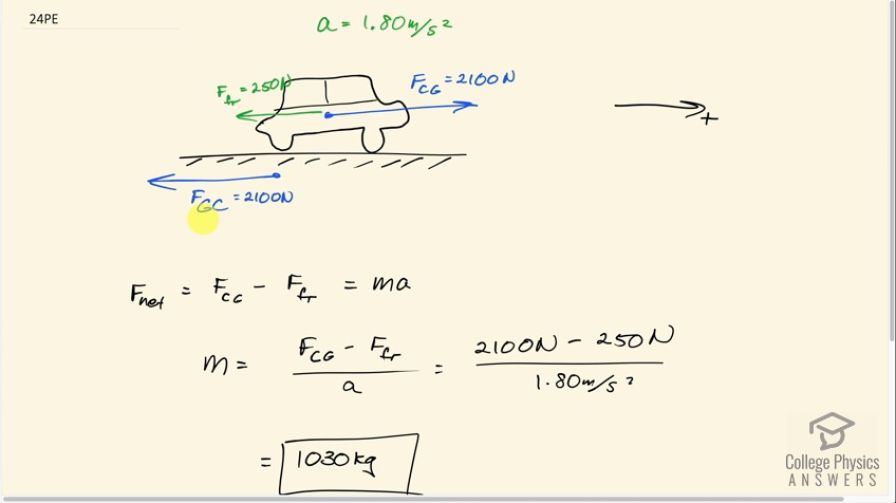
The wheels of a midsize car exert a force of 2100 N backward on the road to accelerate the car in the forward direction. If the force of friction including air resistance is 250 N and the acceleration of the car is , what is the mass of the car plus its occupants? Explicitly show how you follow the steps in the Problem-Solving Strategy for Newton’s laws of motion. For this situation, draw a free-body diagram and write the net force equation.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 4, Problem 24 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
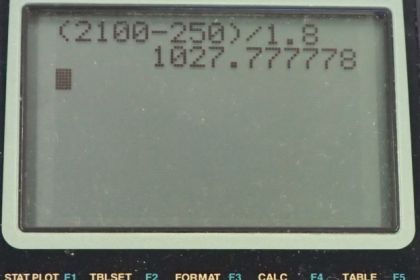
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This car is exerting a force on the ground of 2100 newtons going backwards. So I’ve written subscript g for the force on the ground and c due to the car. And the Newton’s third law counterpart to this, is the force on the car due to the ground. And that's why it’s relevant to tell us what the force on the ground is. Because that's in-turn the force on the car as well, but in the opposite direction. So the force on the car due to the ground is 2100 newtons to the right, and that is the positive direction forwards. And we’re told that air friction is 250 newtons going back. And the overall acceleration is forwards positive 1.80 meters per second squared. We’re going to find out what the with the total mass of the car plus occupants is. And we’ll use the Newton’s second law; which is net force equals mass times acceleration. And the net force is the total forces to the right, which is just one force on the car due to the ground. Minus the total forces to the left, which is that due to friction and we’ll solve for m by dividing both sides by a. So mass is the difference between the force on the car due to the ground and the friction force, divided by acceleration. So that’s 2100 newtons minus 250 newtons all divided by 1.8 meters per second squared. Giving a mass of 1030 kilograms when you round to three significant figures. And I guess if you wanted to be more explicit with the significant figures, you could write 1.03 times ten to the three kilograms. Because written this way there's a bit of ambiguity as to whether this final zero is significant or not. Technically it is not, but sometimes it’s confusing. So this is the most clear way of expressing the number of significant figures.