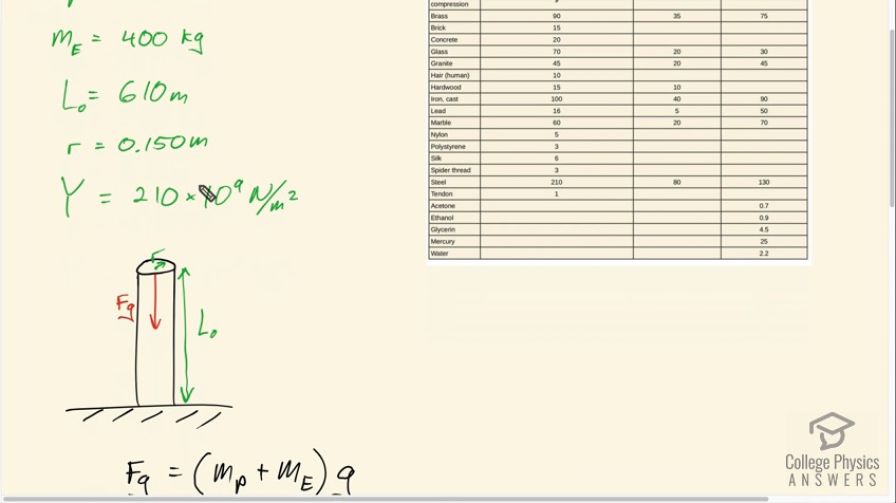
Question
TV broadcast antennas are the tallest artificial structures on Earth. In 1987, a 72.0-kg physicist placed himself and 400 kg of equipment at the top of one 610-m high antenna to perform gravity experiments. By how much was the antenna compressed, if we consider it to be equivalent to a steel cylinder 0.150 m in radius?
Final Answer
Solution video
OpenStax College Physics, Chapter 5, Problem 32 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We have a broadcast antenna with some initial length, L naught, which we're told as 610 meters. A physicist climbs up to the top and brings a 400 kilograms of equipment with him and he weighs 72 kilograms. The total weight of the physicist and equipment is going to be this force of gravity on the top of this cylinder. The cylinder has this given radius of 0.15 meters and we're told to assume it's like a steel cylinder, which means we can look at this data table 5.3 for the elastic moduli, and we see that the Young's modulus for steel is 210 times 10 to the 9 newtons per meter squared. Plug it right here. We have that the change in length of a material that's being compressed, or stretched, is going to be the force applied to one of the ends multiplied by its initial length divided by the Young's modulus times the cross-sectional area of the material. The force is this total gravity of the physicist and the equipment. The area is pi times the radius of the antenna squared. We substitute those two things in until we have the change in length then is 72 kilograms plus 400 kilograms, all times 9.8 newtons per kilogram times 610 meters divided by 210 times 10 to the 9 newtons per square meter, times pi times 0.15 meters squared, which gives a change in length of 0.190 millimeters. Steel is very not compressible.
Comments
Why is the final answer 3 significant figures?
In the video you say to multiply the denominater but in the calculator you divided 210 x 10^9 / pie / 0.15 ^2. Which one should I do?
Hi cvincent, thank you for the question. I think what you're noticing is my personal preference for typing several divides instead of multiplying all the factors in the denominator and then dividing once by that result. In other words, for can be typed into the calculator as "a / b / c / d", which is what I do since the calculator goes from left to right evaluating first "a / b", then dividing that result by "c", and in turn that result by "d". This is the same as "a / ( b x c x d)", which I think is what you were expecting.
Hope that helps,
Shaun


