Question
Two different electrical devices have the same power consumption, but one is meant to be operated on 120-V AC and the other on 240-V AC. (a) What is the ratio of their resistances? (b) What is the ratio of their currents? (c) Assuming its resistance is unaffected, by what factor will the power increase if a 120-V AC device is connected to 240-V AC?
Final Answer
Solution video
OpenStax College Physics, Chapter 20, Problem 81 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
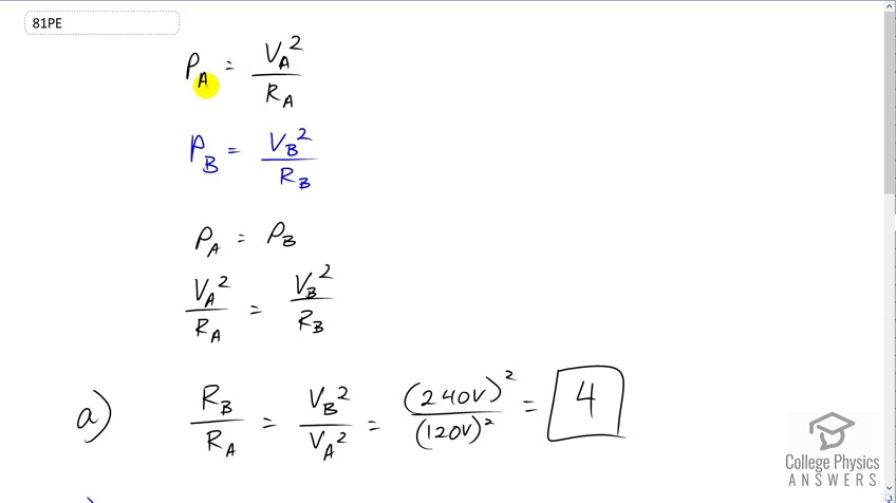
This is College Physics Answers with Shaun Dychko. We consider two electrical devices, one we'll call A and it's meant to be operating at 120 volts. Device B is meant to be operating at 240 volts. The power consumption of device A will be the A's voltage squared, divided by the resistance of device A, and that for B will be the voltage of device B squared, divided by the resistance of device B. Now, the power consumptions are the same we're told. So that means we can equate these two fractions and we do that here. Now we're going to solve for the ratio of their resistances by multiplying both sides by R B over V A squared. The V A's cancel on the left leaving us with R B over R A. On the right hand side the R B's cancel leaving us with V B squared over V A squared. We know what V B and V A are, so we substitute in 240 volts for device B, square that and divide by 120 volts for device A and square that, and we get the number four. This is the ratio of resistance of device B to resistance of device A. So the device B has four times the resistance of device A. That's why it can have the same power consumption despite a higher voltage. Then in part B what is the ratio of their currents? Well, device B has a -- we can express its power consumption in terms of current by going I B squared times R B. For device A, it's when we have A's current squared divided by R A, and this equals one because P B and P A are equal. They consume the same amount of current so dividing equal numbers makes the number one. So we'll solve this for the ratio of current B over current A by multiplying both sides by R A over R B. We get I B squared over I A squared equals R A over R B and then take the square root of both sides. So the ratio I B over I A is the square root of the ratio of resistance A over resistance B. So that's the square root of one over four because we already figured out that R B over R A is four, so that mean the reciprocal is R B over R A which is R A over R B. We can take this to the power of negative one and so that equals four to the negative one since this fraction is the number four, and four to the negative one is one over four. So we're taking the square root of one over four which is one over two. So the current through device B is one half the current through device A. Then in part C, if you take this 120 volt device and put it into the 240 volt circuit, what is going to happen? By what factor will the power increase? Well in the first case, you have the regular 120 volt squared divided by its resistance, and then in the second case you have this new voltage divided by the same resistance because it's the same device. We're going to take the ratio of these two fractions. So the power in the second case, attaching it to the 240 volt circuit, divided by the power in the first case which is when it's attached to the 120 volt circuit is going to be V A two squared over R A. Then multiply by the reciprocal of this because dividing by power A one is the same as multiplying by its reciprocal. The R A's cancel and we're left with V A two squared over V A one squared. So it's the square of the ratio of the voltages. So we have 240 volts squared over 120 volts squared and we get four. So the power consumption of this device meant for a 120 volts circuit will increase by a factor of four when it's attached to a circuit at 240 volts.