Question
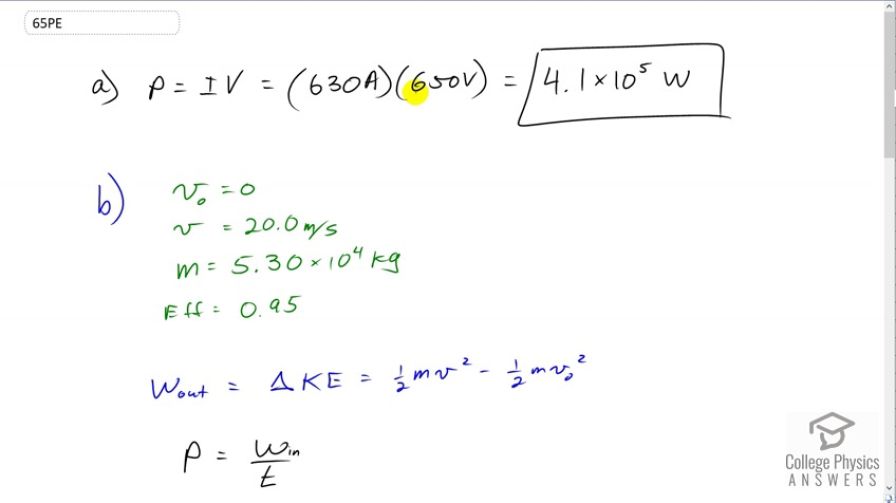
A light-rail commuter train draws 630 A of 650-V DC electricity when accelerating. (a) What is its power consumption rate in kilowatts? (b) How long does it take to reach 20.0 m/s starting from rest if its loaded mass is , assuming 95.0% efficiency and constant power? (c) Find its average acceleration. (d) Discuss how the acceleration you found for the light-rail train compares to what might be typical for an automobile.
Final Answer
- . The car accelerates faster.
Solution video
OpenStax College Physics, Chapter 20, Problem 65 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The power consumption of this train will be the current that it draws multiplied by the voltage. So that’s 630 Amps multiplied by 650 Volts which is 4.1 times ten to the five watts. And then we are asked to figure out how long does it take to reach 20 meters per second starting from rest. And knowing that its mass when it’s fully loaded is 5. 3 times ten to the four kilograms. And assuming the efficiency of 0.95. So that means 0. 95 multiplied by the power input turns into kinetic energy. So, we’ll get to that in a second. So we have the resulting work output as the change in kinetic energy, so it’s one half mass times final velocity squared minus one half mass times initial velocity squared. But it starts from rest and so there is only as one term here. And we also that power that’s consumed is the work in per time. And so we can figure out what this work input is that’s consumed from the electrical utility by multiplying the power by time. Now efficiency is the work output divided by the work input. And so we solve this for work output because we have another expression for work output and so we create the two. And, so it’s multiplied both sides by work input here. And we have work output is efficiency times work input and the work input, we’ve already said is power times time. And so we make that substitution here. And then this is the expression for work output. And we equate it to the changing kinetic energy. So we have efficiency times power times time equals one half mass times final velocity squared. And we solve this for t by dividing both sides by efficiency times power. And we get time is mv squared over two times efficiency times power. And that is 5.3 times ten to the four kilograms times 20 meters per second squared, divided by two times 0.95 times 4.095 times ten to the five watts. And that gives 27 seconds. So that’s how many seconds it will take to get from zero to 20 meter per second. Then, we figure out what is acceleration is and that’s final velocity will be the initial velocity plus the acceleration times time. And we can solve this for a by subtracting v naught for both sides. And then dividing both sides by t. We got a is final velocity minus initial divided by time. So it’s 20 meters per second minus zero divided by 27.248 seconds. And that gives 0.73 meters per second squared. And then part d comparing that to a typical car. A car can usually get to 100 kilometers per hour in about ten seconds. And so in meters per second that final speed is times by 1000 meters per kilometer times one hour for every 3600 seconds. And you end up with 27.8 meters per second. So, the acceleration of the car is 27.8 over ten seconds which gives 2.8 meters per seconds squared. And so the car accelerates quite a bit faster than a train. But the train has the advantage that since it’s on a straight track which is usually not interrupted, meaning there’s no intersections going across the track quite often. You know, depending on the type of train, it can reach a faster final speed. And so, the train can ultimately get you to a destination more quickly maybe.

