Question
(a) Digital medical thermometers determine temperature by measuring the resistance of a semiconductor device called a thermistor (which has ) when it is at the same temperature as the patient. What is a patient's temperature if the thermistor's resistance at that temperature is 82.0% of its value at (normal body temperature)? (b) The negative value for may not be maintained for very low temperatures. Discuss why and whether this is the case here. (Hint: Resistance can't become negative.)
Final Answer
a)
b) See video solution for explanation.
Solution video
OpenStax College Physics, Chapter 20, Problem 37 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
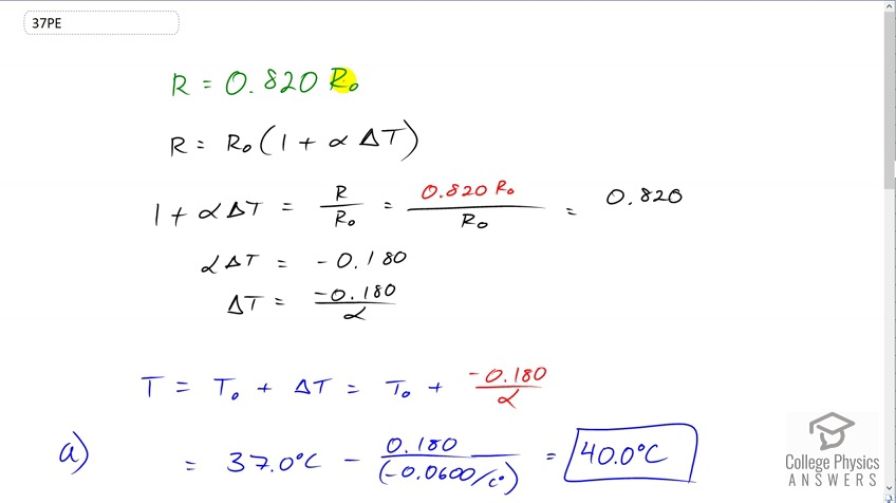
This is College Physics Answers with Shaun Dychko. We're told that the resistance of the thermistor is 82 percent of what it was originally at 37 degrees. So here's our formula for resistance as it depends on temperature. So resistance will be the original resistance times one plus the temperature coefficient of resistivity times the change in temperature. We can solve this for change in temperature by first dividing both sides by R naught and we get this line, and then we substitute in 0.82 R naught in place of R because we're told the resistance is 82 percent of what it was. Then the R naughts cancel leaving us with 0.82 on the right hand side. Then we subtract one from both sides and we get alpha times delta t is negative 0.180. So divide both sides by alpha, and we get change in temperature is negative 0.180 over alpha. Now the new temperature is going to be the original temperature plus this change in temperature that we just found. So we substitute in for delta t here and so the new temperature will be t naught plus negative 0.180 over alpha. That is 37.0 degrees Celsius minus 0.180 over the temperature coefficient of resistivity for a thermistor which is negative 0.06. That gives 40.0 degrees Celsius. So that's what the LCD display of the thermometer should read. Now, in order for the resistance to not be negative because a resistance that's negative doesn't make sense, this factor here has to be greater than zero. So we can see that this alpha, this temperature coefficient of resistivity can't be negative all the time. There's going to have to be some temperature dependence of this factor as well. So things get complicated. So not only does resistance depend on this times change in temperature, but this in turn also depends on the temperature and so the reality is probably a bit more complicated than what this equation shows. But maybe this equation is good for small changes in temperature. So this has to be greater than zero and in this particular question we have the final temperature minus 37. So all of this has to be greater than negative one after you subtract one from both sides and then to figure out restrictions on alpha we have to divide both sides by t minus 37.0 degrees Celsius. Now the thing is we don't know whether t is greater or less than 37 so we don't know whether we're dividing by a negative number. If we're dividing by a negative number we need to flip this inequality. When you multiply or divide both sides by a negative, with an equality you have to switch the sign of it. So if t is less than 37, then the inequality gets flipped to be less than. So alpha has to be less than one over 37 minus t. If t is greater than 37 then we would leave the inequality as it is because we're multiplying and dividing by a positive. So we have alpha is greater than negative one over t minus 37 and just to make it look a little nicer I'll multiply top and bottom by negative one. So we have one over 37 minus t and in this particular question, the final temperature is 40 and so alpha has to be more than negative 0.33 which it certainly is because it is negative 0.06 which is a lot more than negative 0.33. There we go!
