Question
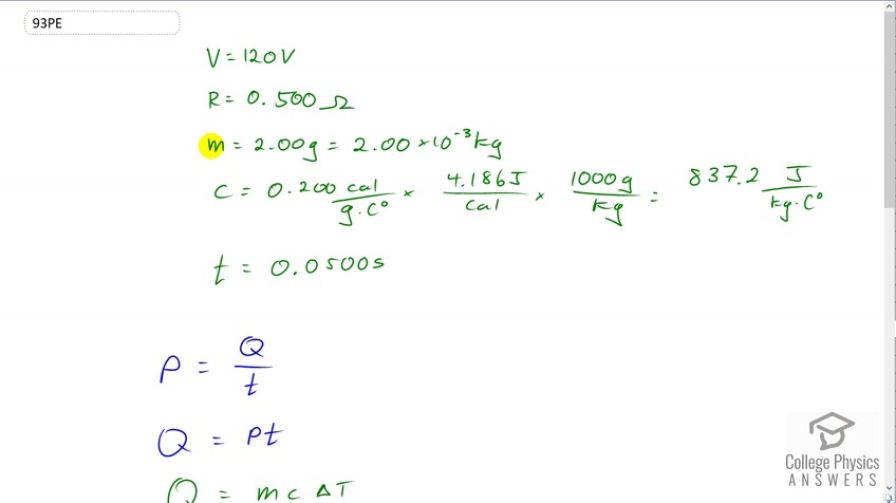
A short circuit in a 120-V appliance cord has a resistance. Calculate the temperature rise of the 2.00 g of surrounding materials, assuming their specific heat capacity is and that it takes 0.0500 s for a circuit breaker to interrupt the current. Is this likely to be damaging?
Final Answer
Yes, this would likely be damaging as it could melt or ignite the materials.
Solution video
OpenStax College Physics, Chapter 20, Problem 93 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We have short circuit involving a voltage of 120 Volts. And the short circuit has a resistance of 0.5 ohms. And there are two grams of material surrounding the short circuit, which is 2.00 times 10 to the minus three kilograms. And the specific heat of the material that is surrounding is 0.2 calories per gram per Celsius degree and we’ll convert that into Joules per kilogram Celsius degree because those are the units we need for the formulas. So we multiply by 4.186 Joules per calorie and then times 1000 grams per kilograms. And then that cancels away the units we don’t want, leaving us with units that we do want. So that’s 837.2 Joules per kilograms Celsius degree. And the time before the circuit breaker stops the short circuit is 0.05 seconds. So, the power output of the short circuit is the energy that is going to go heating this running material per time. And that energy is going to be the power multiplied by time. And that energy is also going to be the energy that causes a temperature rise in the material. And this formula comes from Chapter 14 if you need to review that. This is mass multiplied by specific heat multiplied by change in temperature. So we equate these two things together because they are both equal to Q. And so we have mc delta T equals power times time. And so we find the temperature rise by dividing both sides by mc. And we get the temperature rise as the power times time divided by mass times specific heat. And the power is going to be the voltage squared divided by resistance. And so we substitute that in for P. And so we have 120 Volts squared times 0.05 seconds of time before the circuit breaker stops the circuit divided by 0.5 ohms resistance of the circuit times two times ten to the minus three kilograms times 837.2 Joules per kilograms per Celsius degree. And this gives us 860 Celsius degree of temperature rise. And that likely would be damaging, that can probably either melt the material that surrounding it or ignite it.
