Question
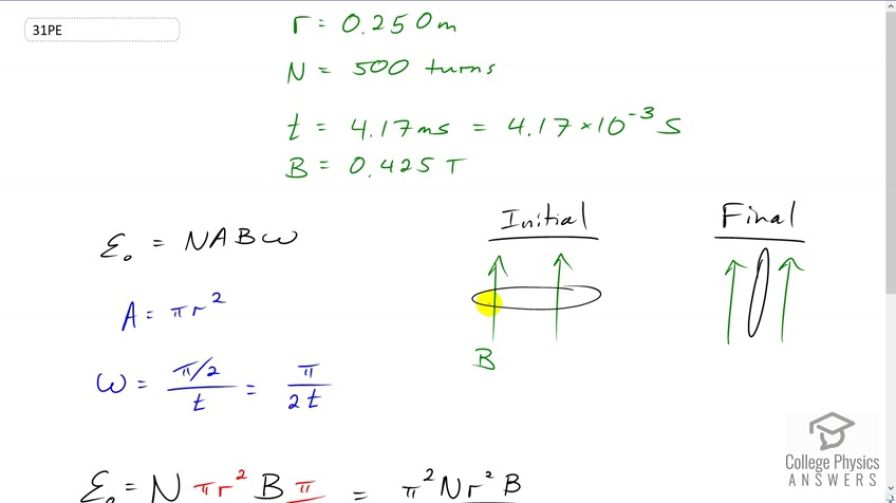
What is the peak emf generated by a 0.250 m radius, 500-turn coil is rotated one-fourth of a revolution in 4.17 ms, originally having its plane perpendicular to a uniform magnetic field of strength 0.425 T. (This is 60 rev/s.)
Final Answer
Solution video
OpenStax College Physics, Chapter 23, Problem 31 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A circular coil with 500 turns is rotated one quarter of a revolution in the presence of a magnetic field where initially the field is going through the coil, plane of the coil perpendicular. And then, it becomes parallel. So, that makes this one quarter of a revolution. And, the field strength is 0.425 Tesla and the radius of this coil is 0.25 meters. And, we're told that the time it takes to do one quarter of a revolution is 4.17 milliseconds, which we'll write as 4.17 times 10 to the minus 3 seconds. And, the question is what is the peak voltage produced in this scenario? So, that's going to be the number of turns in the coil times its cross-sectional area times the magnetic field strength times the angular velocity. Now, we'll have to do substitutions for both the area and Omega. So, the area is going to be Pi times radius squared, and then the angular velocity we have to express this angle in radians. This Omega is in radians per second. And so, we have Pi over 2 radians is the angle in radians for a quarter turn. And, we divide that by the time t. And so, this works out to Pi over 2 t. So, you can also say this is a rotation of 90 degrees and then know that your angling radians is 90 degrees times Pi radians for every 180 degrees, you could say that. And then it works out to Pi over 2. So, when we place Omega with Pi over 2 t, and then replace area with Pi r squared and we end up with peak voltage as Pi squared times number of turns times the radius squared times the magnetic field strength divided by 2 times time. So, that's Pi squared times 500 times 0.25 meters squared times 0.425 Tesla divided by 2 times 4.17 times 10 to the minus 3 seconds, which gives 15.7 kilovolts will be the peak voltage.
