Question
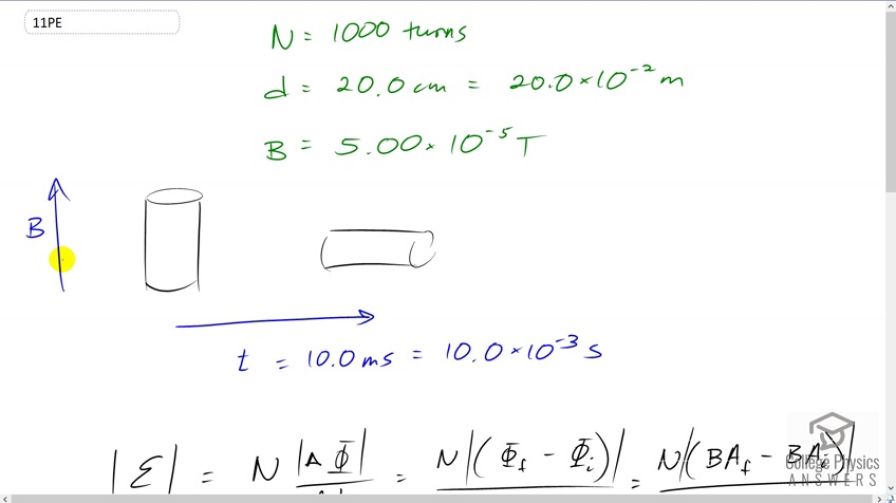
An emf is induced by rotating a 1000-turn, 20.0 cm diameter coil in the Earth’s magnetic field. What average emf is induced, given the plane of the coil is originally perpendicular to the Earth’s field and is rotated to be parallel to the field in 10.0 ms?
Final Answer
Solution video
OpenStax College Physics, Chapter 23, Problem 11 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A coil with 1000 turns is initially having its plane perpendicular to the earth's magnetic field, which we'll suppose is straight up here. And then it's turned a quarter of a turn in ten milliseconds. And then its plane will be parallel to the earth's magnetic field. So, the earth's magnetic field is going up and the plane of this coil is also up and down here. So, the question to figure out the induced EMF is we need to know by how much has the magnetic flux changed and we take that and divide it by the amount of time during which it changed. And then, multiply that by the number of turns in this coil. So, the induced EMF, we don't really care about the direction of it, so we'll put the absolute value signs around it. And, it's going to be N times change of flux over change in time and that is the final flux minus the initial flux and I'm putting absolute value signs around that because we don't care about the sign. And, the final flux will be the magnetic field strength of the earth multiplied by the final area through which the field lines cross minus the magnetic field strength of the earth times initial area through which it crosses. Now, the final area through which the field lines cross is zero because the field lines are parallel to the plane of the loop here and so, none of them cross through the loop. And so, this term is zero. And so, the induced EMF is going to be the number of turns times the magnetic field strength times the initial area divided by time. So, the area is pi times radius squared, and we're given the diameter, so we'll substitute diameter divided by two in place of R, and that makes pi D squared over four. So, we'll plug that in for A initial. And then, induced EMF then is going to be 1000 turns times the magnetic field strength due to the earth, which is five times ten to the minus five Tesla times pi times 20 times ten to the minus two meters, that's 20 centimeters squared divided by four times ten times ten to the minus three seconds. This is ten milliseconds. This makes 157 milliVolts induced EMF.
Comments
again, wrong. you again divided when you needed to multiply. This happens way too much
Please see my comment here for an explanation of why there are no parentheses surrounding the denominator to enclose factors multiplied in the denominator.


