Question
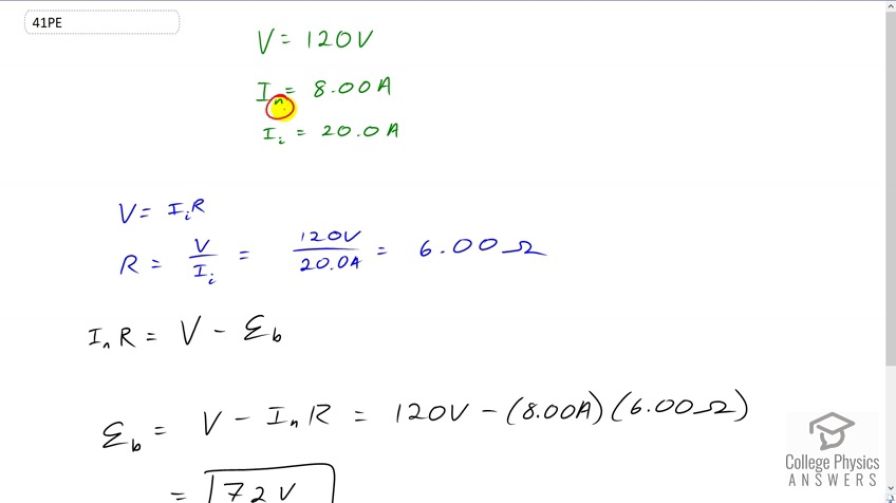
What is the back emf of a 120 V motor that draws 8.00 A at its normal speed and 20.0 A when first starting?
Final Answer
Solution video
OpenStax College Physics, Chapter 23, Problem 41 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. An electric motor is connected to 120 volts. And when it's running at its regular speed its normal operating speed it's drawing eight amps of current and initially before it starts turning when it's just switched on. It has a current of 20 amps going through it. And so when it's just switched on there's no back EMF because it isn't turning it. And so we can use this information to calculate the resistance of the armature and then knowing that resistance will figure out the back EMF in the next step. So first of all let's figure out resistance by saying that the voltage of the source equals the initial current times resistance. And then we'll divide both sides by initial current. And then R equals V over I. So it’s 120 volts divided by 20 amps which is six point zero zero ohms. Now sometime later after it gets up to its normal operating speed the normal current multiplied by resistance is gonna be the difference between the voltage of the source and the back EMF that is produced. So with this back EMF we’ll take away from the voltage and it's going to result in a lower current. And so we can solve for the back EMF by adding it to both sides and then also subtracting normal current times resistance from both sides and then we have Back EMF as voltage minus normal current times resistance. So that's 120 volts minus eight amps times six ohms which gives 72 volts is the back EMF.
