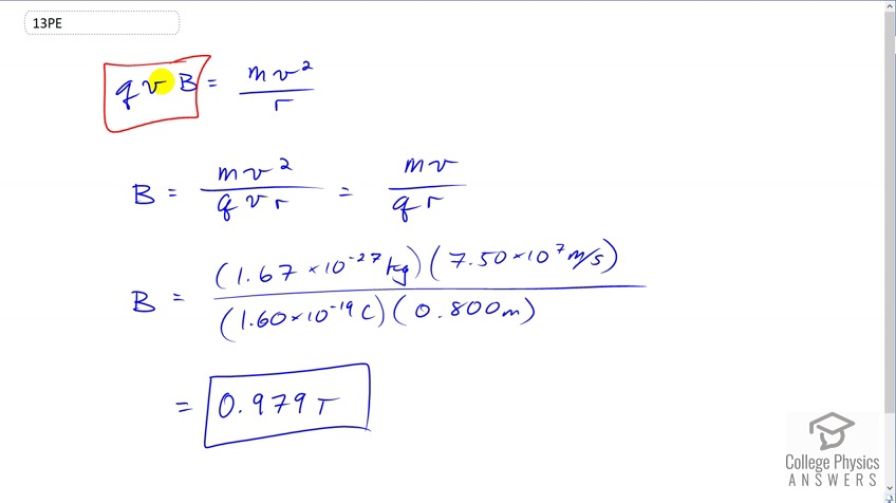
A proton moves at $7.50\times 10^7\text{ m/a}$ perpendicular to a magnetic field. The field causes the proton to travel in a circular path of radius 0.800 m. What is the field strength?

Video Transcript

This is College Physics Answers with Shaun Dychko. The force due to the magnetic field on the charge is the charge times its speed times the magnetic field strength. This is assuming the charge velocity is perpendicular to the magnetic field which it is, in this question. And that magnetic force is going to be providing the centripetal force of the charge needs to go in a circle. And so the centripetal force is the mass of the charge times its speed squared divided by the radius of the circle. So we can solve this for B by dividing both sides by qv. And we get that the magnetic field is mv squared over qvr. And so one of the v is cancel and we end up with mv over qr. So the magnetic field strength is the mass of the proton which is 1.67 times ten to the minus 27 kilograms times the speed which is 7.5 times ten to the seven meters per second divided by the charge on the proton which is the elementary charge 1.6 times ten to the minus 19 Coulombs times the radius of the path which we're told is 0.8 meters, giving us magnetic field strength of 0.979 Tesla.