Question
(a) A cosmic ray proton moving toward the Earth at experiences a magnetic force of . What is the strength of the magnetic field if there is a angle between it and the proton’s velocity? (b) Is the value obtained in part (a) consistent with the known strength of the Earth’s magnetic field on its surface? Discuss.
Final Answer
- This is 60% of the magnetic field strength of the Earth, and, since the Earth's magnetic field varies with position around the planet, yes, this answer is consistent with the Earth's magnetic field.
Solution video
OpenStax College Physics for AP® Courses, Chapter 22, Problem 9 (Problems & Exercises)

vote with a rating of
votes with an average rating of
.
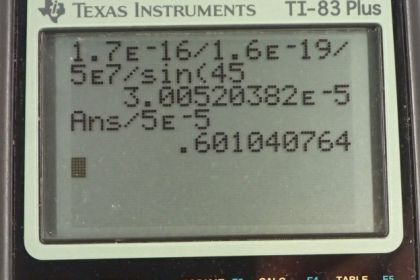
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The force on a charge moving in the presence of a magnetic field is the charge in Coulombs times its velocity in meters per second times the magnetic field strength times sine of the angle between the velocity of the magnetic field. And we can solve for B by dividing both sides by qv sine theta. And so the magnetic field strength is the force divided by qv sine theta. So that's 1.7 times ten to the minus 16 newtons divided by the elementary charge which is the charge on a proton, because we are told that this cosmic array consist of a proton. So that's 1.6 times ten to the minus 19 Coulombs times five times ten to the seven meters per second times sine 45 which is the angle between the velocity of the magnetic field. This gives a magnetic field strength of 3.01 times ten to the minus five Tesla. Now, the magnetic field strength of the earth is about five times ten to the minus five Tesla. And I say approximately equal because this magnetic field strength really varies depending on where your position is around the earth. And so we'll take the magnetic field we determined in part a and this is part b. And we'll take the magnetic field we determined in part a and divided by magnetic field of the earth in order to compare them. And we have three times ten to the minus five divided by five times ten to the minus five which is 0.60. So this means that our magnetic field that we calculated is 60 percent that of what is generally accepted to be the field strength of the earth and since this number is approximately can change, depending where you are. This means yes, our answer in part a is consistent with the earth's magnetic field.