Question
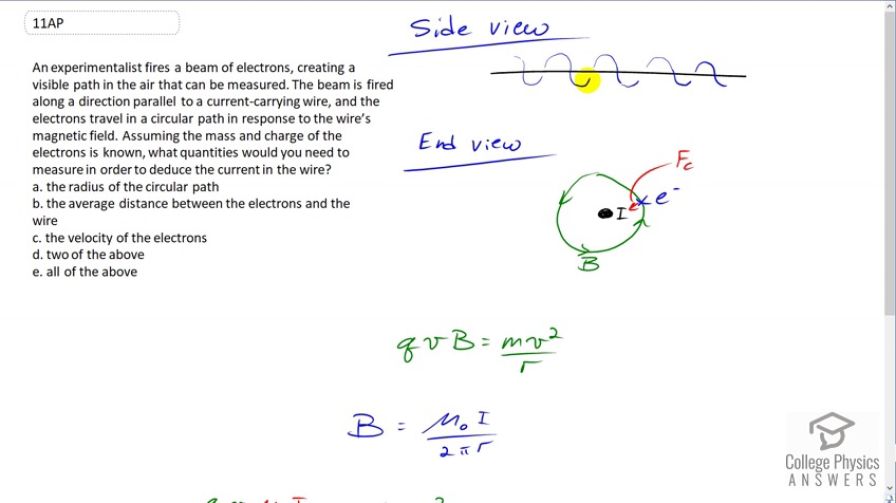
An experimentalist fires a beam of electrons, creating a visible path in the air that can be measured. The beam is fired along a direction parallel to a current-carrying wire, and the electrons travel in a circular path in response to the wire’s magnetic field. Assuming the mass and charge of the electrons is known, what quantities would you need to measure in order to deduce the current in the wire?
- the radius of the circular path
- the average distance between the electrons and the wire
- the velocity of the electrons
- two of the above
- all of the above
Final Answer
(c)
Solution video
OpenStax College Physics for AP® Courses, Chapter 22, Problem 11 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. Here is a side view of the wire in black with this electron beam traveling in a spiral around the wire. So electrons are going this way but they're going in a circle around the wire as they do that. Here is an end view. Oh by the way I should draw the direction of the current in the wire. Current is going to the left. Here's an end view -- I'll explain why the current is going to the left by talking about the end view picture. We have a current coming out of the page which I'm drawing with a dot here, and this electron is going into the page which I've drawn with an x. We use the right hand rule to determine the direction of force on this electron. There's a couple ways to compensate for the fact that this is an electron and not a proton when you're using the right hand rule. I'm going to point my thumb in the opposite direction to its velocity. So I'm going to point the thumb out of the page and then point my fingers in the direction of the magnetic field and then the palm is pointing in the direction of the force on it. You could have alternatively put your thumb in the direction of the velocity which is into the page, again with fingers pointing in the direction of the magnetic field and then taken the force direction to be the opposite to the direction that your palm is pointing. That would also work too. Either way, you get a force directed towards the wire which is the center of curvature of its path. This is the centripetal force. Now the centripetal force is mass of the electron times its velocity squared, divided by the radius of its circular path. That is going to be provided by this magnetic force which is the charge times its speed, times magnetic field strength. Now, this velocity and this magnetic field are perpendicular here and so we can just use q v B with no angle here. Now the magnetic field due to a long current-carrying wire is permeability of free space times the current, divided by two pi, times the distance from the wire. Now this distance from the wire is the same as the radius of curvature of the electron's path. So we can use the same letter r there. So we'll substitute for B with this expression. So we write q v mu naught I over two pi r equals m v squared over r. Then when you solve for I by multiplying both sides by two pi r over q v mu naught, we see a few things happen. Number one -- just a second, let's write this down. Number one is we see that the r's cancel. So surprisingly it's not important to know what the radius of the circular path is. One of the v's cancel and we're left with this expression. So the current in the wire is going to be two pi times the mass of the electron, times its speed, divided by mu naught times q. Now the question tells us that we know what the mass and the charge are, so we know m and q. Of course we know what pi and mu naught are, since those are constants. That means the only thing we need to know is the speed of the electrons. So the answer is C, the velocity of the electrons.