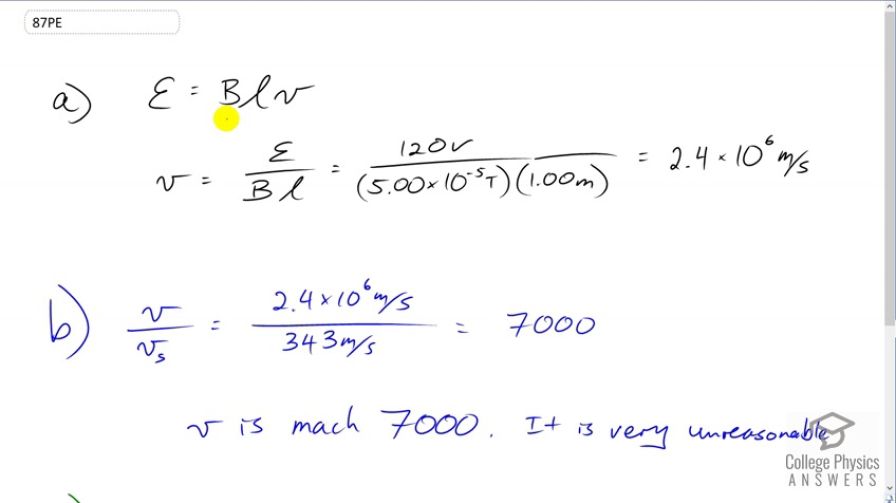
An inventor wants to generate 120-V power by moving a 1.00-m-long wire perpendicular to the Earth’s $5.00\times 10^{-5}\text{ T}$ field. (a) Find the speed with which the wire must move. (b) What is unreasonable about this result? (c) Which assumption is responsible?

Video Transcript

This is College Physics Answers with Shaun Dychko. The EMF that would be induced in this wire is given by this Hall Effect formula, the voltage is the magnetic field strength times the length of the wire times its speed, this assumes that the velocity and magnetic field are perpendicular. So we can solve for v by dividing both sides by BL, and we get v is the voltage divided by magnetic field strength times length, so that’s 120 Volts divided by five times ten to the minus five Tesla times one meter, which is 2.4 times ten to the six meters per second. This is an unrealistically large speed, because if you divide it by the speed of sound, you see that it’s 7000 times the speed of sound, sound goes at 343 meters per second or so. So this is mach 7000, and that’s very unreasonable, a fighter jet can go mach five, maybe. And so expecting such a high voltage from only a single wire in such a small magnetic field that the Earth has is what’s unrealistic.