Question
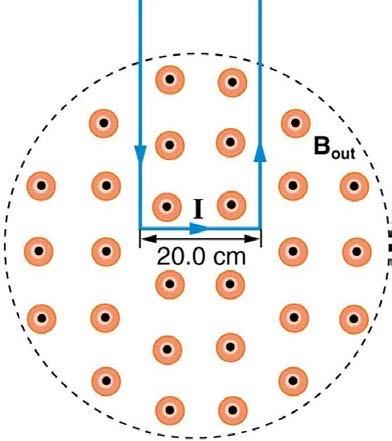
Measurements affect the system being measured, such as the current loop in Figure 22.62. (a) Estimate the field the loop creates by calculating the field at the center of a circular loop 20.0 cm in diameter carrying 5.00 A. (b) What is the smallest field strength this loop can be used to measure, if its field must alter the measured field by less than 0.0100%?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 22, Problem 67 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
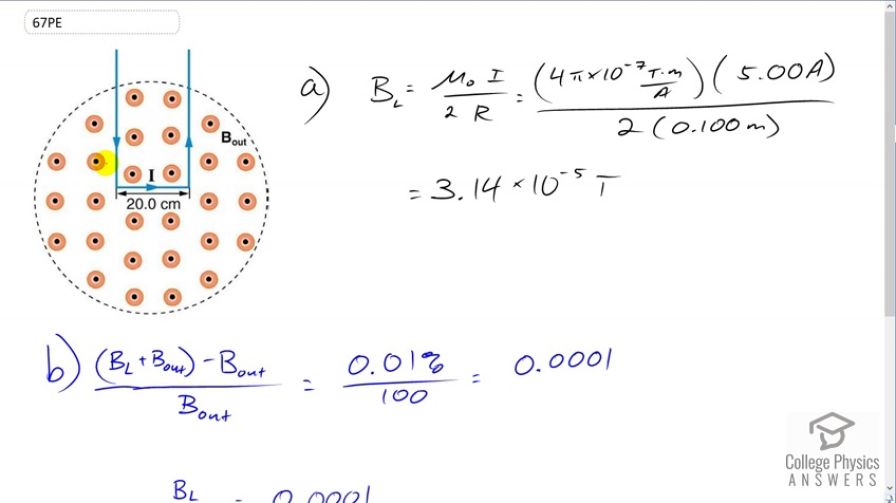
This is College Physics Answers with Shaun Dychko. This question asks us to imagine that we have this loop here acting as a sensor for an external magnetic field. It's called, labeled B out. We're told here to imagine that the loop is actually circular because we have a formula that will calculate the magnetic field inside such a loop. So the picture is actually more like this where we have a current going down one side of this wire here and then up the other wire. But those wires are right beside each other and then there's a circle at the end instead of a rectangle like this. The radius of the circle is meant to be 0.1 meters. So we'll calculate the magnetic field that will be produced by a five amp current going through this loop and then we'll say, well, when we place this loop into an external magnetic field that we want to measure, we want to be sure that we don't change that field by more than 0.01 percent because this loop with this current going through it will produce a field of its own. Because of that it's going to alter the field that it's in the presence of and that's going to have an effect on the measurement. So the magnetic field inside the loop will be the permeability of free space times current divided by two times its radius. So that's four pi times ten to the minus seven Tesla meters per amp, times five amps over two times 0.1 meters, which is 3.14 times ten to the minus five Tesla. Now when this loop is placed in this external field, the total field that results the loop's field plus the external field, minus the external field, this difference which is the amount by which the loop's field is affecting the external field, that difference divided by the external field has to be 0.01 percent at the most. So we'll divide that by 100 to turn it into a decimal. So that's 0.0001 and B L plus B out minus B out just makes B L because these are going to make zero. So we have B L over B out equals 0.0001 and we're solving for the external field, maximum external field, the smallest external field, such that the loop's field changes it by 0.01 percent. So, we have the field is the loop's field divided by 0.0001. So that's 3.14 times ten to the minus five Tesla over 0.0001 which is 0.314 Tesla. So this is the smallest field that you can measure with a loop and change it by only this much. There we go!