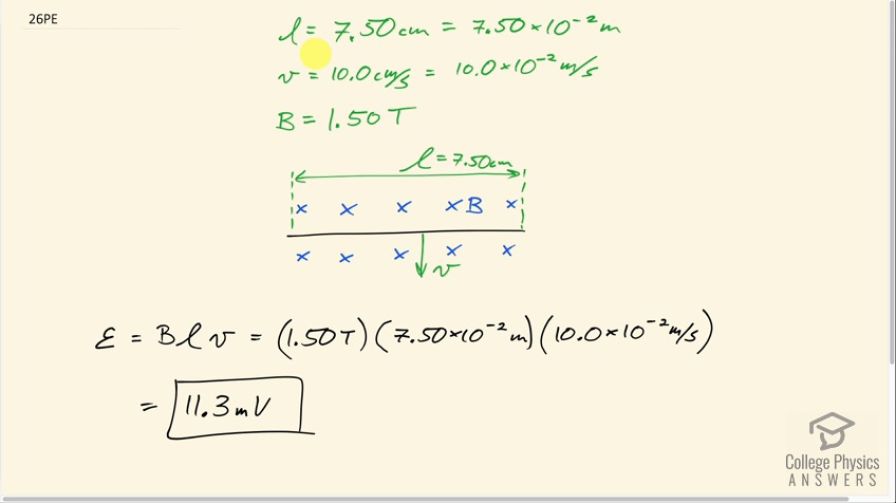
Calculate the Hall voltage induced on a patient’s heart while being scanned by an MRI unit. Approximate the conducting path on the heart wall by a wire 7.50 cm long that moves at 10.0 cm/s perpendicular to a 1.50-T magnetic field.

Video Transcript

This is College Physics Answers with Shaun Dychko. We are going to estimate the Hall voltage induced in a patient's heart while being scanned by a MRI unit. So we are told to imagine that their heart is like this wire 7.50 centimeters long, which I convert into meters by multiplying by 10 to the minus 2 moving at a speed of 10.0 centimeters per second which is 10.0 times 10 to the minus 2 meters per second and the magnetic field strength is a very large 1.50 tesla; it's a small number 1.50 but that field strength is larger than any permanent magnet can ever do and it's possible in the MRI machine only because of superconducting electromagnets. So the Hall voltage would be the magnetic field strength multiplied by the length of the wire times the speed and this velocity and the magnetic field are perpendicular so we don't need to write sin Θ although you could if you wanted and do sin of 90, which would be 1. So we have 1.50 tesla times 7.50 times 10 to the minus 2 meters times 10.0 times 10 to the minus 2 meters per second, this works out to 11.3 millivolts. And we expect this answer to be small because if there was a large induced voltage then that would be potentially harmful to the person. So people get MRI's all the time so it must be that this induced voltage is really small.