Question
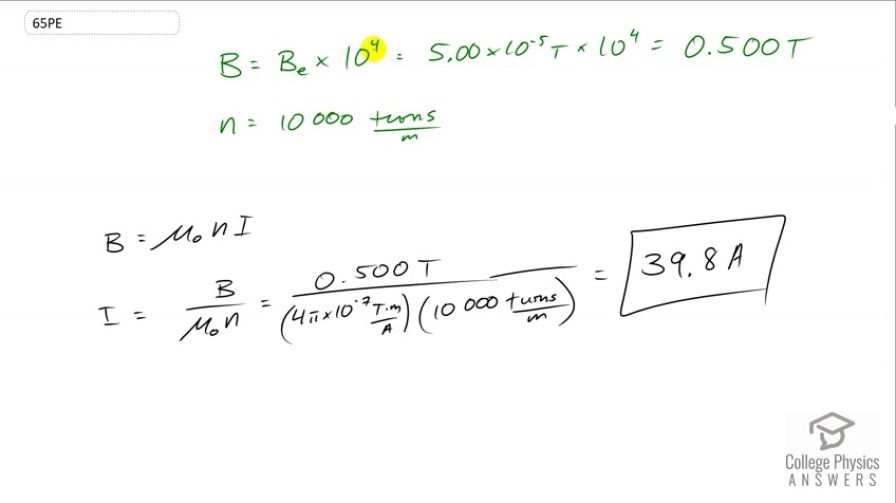
What current is needed in the solenoid described in Exercise 22.58 to produce a magnetic field times the Earth’s magnetic field of ?
Final Answer
Solution video
OpenStax College Physics, Chapter 22, Problem 65 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. In this question we have a solenoid with ten thousand turns per meter and the magnetic field inside is meant to be ten to the four times the magnetic field due to the earth. So the earth has a field of about five times ten to the minus five Tesla, so times by ten to the four gives us 0.500 Tesla. So the magnetic field inside the solenoid is the permeability of free space, assuming there's just nothing inside the solenoid, times the number of turns per meter, times the current. We'll solve this for I by dividing both sides by mu naught n. So I is magnetic field over mu naught n. So that's 0.5 Tesla divided by four pi times ten to the minus seven Tesla meters per amp, times ten thousand turns per meter, giving us 39.8 amps of current.
Comments
wrong
Hi Jodaniel, I like to get them right... ;) Could you please be more specific on what you believe is wrong? Do you see where the mistake is?
All the best,
Shaun
Please see my comment here for an explanation of why there are no parentheses surrounding the denominator to enclose factors multiplied in the denominator.


