Question
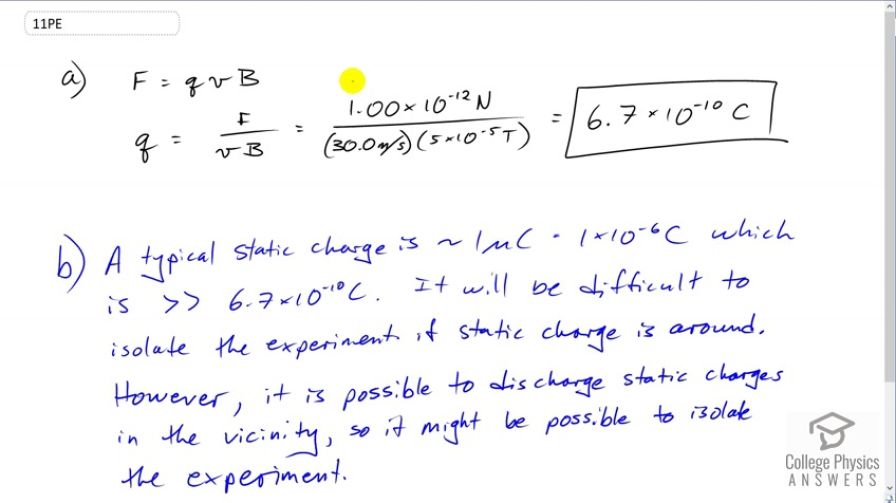
(a) A physicist performing a sensitive measurement wants to limit the magnetic force on a moving charge in her equipment to less than . What is the greatest the charge can be if it moves at a maximum speed of 30.0 m/s in the Earth’s field? (b) Discuss whether it would be difficult to limit the charge to less than the value found in (a) by comparing it with typical static electricity and noting that static is often absent.
Final Answer
- A typical static charge is about which is much greater than . It will be difficult to isolate the experiment if static charge is around. However, it is possible to discharge the static charges in the vicinity, so it might be possible to isolate the experiment after all. Electronics manufacturing facilities have probably created systems and techniques for removing static charges that would otherwise affect their manufacturing processes.
Solution video
OpenStax College Physics, Chapter 22, Problem 11 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The physicist wants to isolate their experiment so that their charge that's moving in their experiment experiences a force of only one times ten to the minus 12 newtons at the most due to the Earth's magnetic field. And so, we'll see what the maximum charge this could be by dividing both sides of this formula by vB. This is the force due to the magnetic field on a moving charge. And so we have that q is the force divided by the speed of the charge times the Earth's magnetic Field B. So that's one times ten to the minus 12 newtons divided by 30 meters per second times five times ten to the five Tesla, giving us 6.7 times ten to the minus ten Coulombs. Now, a typical static charge is about a micro Coulomb which is one times ten to the minus six which is much much larger than this. So, if there is a static charge around, it will probably go on to the thing that's moving in her experiment and make it have a charge on the order of micro Coulombs which would ruin the experiment because that would exceed this charge here. However, it is possible to discharge static charges. I mean, when you touch a door knob after rubbing your feet on the carpet and you see a spark. What you're doing is the charge is moving from one object to the other and the static charge is being equalized or the charge is being equalized on both things. So there's no net static charge anymore. The charge has been discharged, so to speak. It's possible to do that in her experiment and static charges don't just show up really by accident very easily. You have to do some rubbing to make some static charge often. So, it should be possible to isolate the experiment and in a computer chip manufacturing facility, static charges would be a real concern. And so I'm sure that they've developed a good technology to neutralize any static charges. You know, maybe there is really humid air because the polar water molecules in the air will neutralize static charges and when you buy computer electronics, they often come in a static proof bag, a little translucent brown paper plastic bag. Anyway, I think she'll succeed and she doesn't have to take some steps to address the static charges here because if she does nothing, a static charge would probably exceed the limit of what's possible in her experiment.
