Question
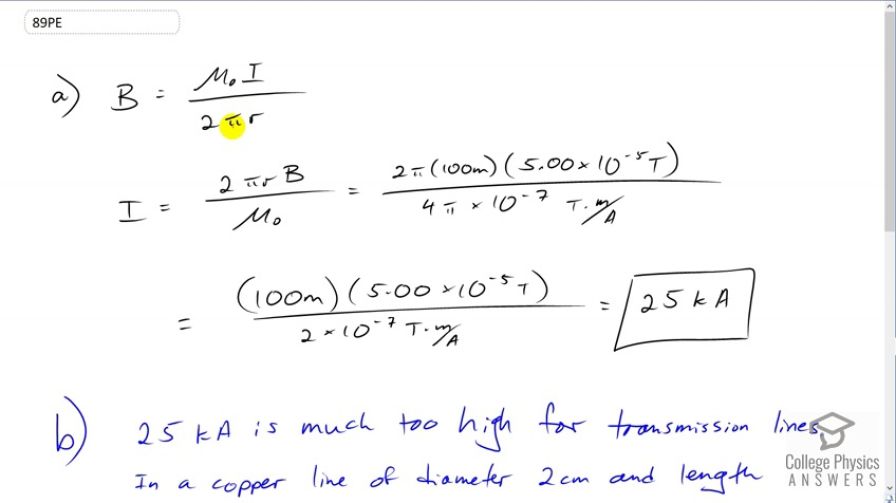
A surveyor 100 m from a long straight 200-kV DC power line suspects that its magnetic field may equal that of the Earth and affect compass readings. (a) Calculate the current in the wire needed to create a field at this distance. (b) What is unreasonable about this result? (c) Which
assumption or premise is responsible?
Final Answer
- This would result in a power loss of in copper transmission lines with a diameter of 2 cm and a length of 100km. This power loss is completely uneconomical.
- The distance of 100 m is too far from the power line.
Solution video
OpenStax College Physics, Chapter 22, Problem 89 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The magnetic field strength from a long current-carrying wire is the permeability of free space times the current in the wire divided by 2 pi times the distance from the wire. So we’ll solve for I by multiplying both sides by 2 pi r over mu naught, so the current is 2 pi r B over mu naught, so that’s 2 pi times 100 meters times five times ten to the minus five Tesla divided by 4 pi times ten to the minus seven Tesla meters per Amp, and I like to cancel the 2 pi with one of the twos there and the pi, leaving us with two times ten to the minus seven on the bottom. This works out to 25 kiloAmps, and that is much too high of a current for a transmission line. If we assume that we have a copper transmission line of diameter say two centimeters and a length of maybe 100 kilometers which is actually a conservative estimate for the distance between a power generating station and the city that it’s delivering the power to. The resistance on this line would be given by the resistivity of copper times the length divided by the cross sectional area, so that’s 1.72 times ten to the minus eight which is in units of Ohm meters, times 100 times ten to the three meters divided by pi times the diameter squared over four, this gives about 5.5 Ohms. The reason I calculated this resistance is because we can figure out the power that’s lost in these transmission lines to just heat dissipated into the environment around the lines as the current squared times resistance of these lines. So the current of 25 kiloAmps, we square that, multiply by 5.5, and we get 3.4 GigaWatts. And that is definitely not economical to be losing that much power in transmission line. So the distance of 100 meters is too far from the power line, going much closer than 100 meters would result in a smaller, more realistic current.
