Question
What angle would the axis of a polarizing filter need to make with the direction of polarized light of intensity to reduce the intensity to ?
Final Answer
Solution video
OpenStax College Physics, Chapter 27, Problem 87 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
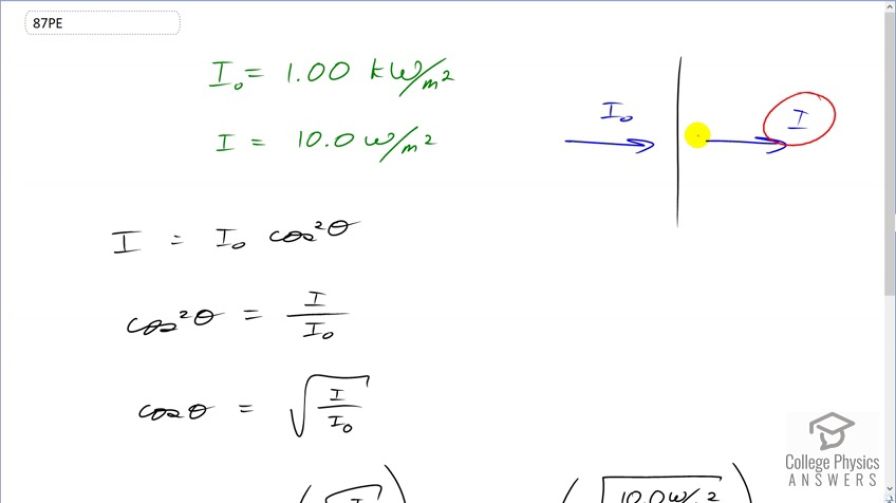
This is College Physics Answers with Shaun Dychko. Polarised light is incident on a polarizing film which then transmits some intensity I and the angle between the polarization axis and the polarization direction of the incident light is what we have to find. And so we have an expression here that the transmitted intensity equals the incident intensity multiplied by a cosine of the angle between the polarizations squared and so we're gonna solve for this theta. Because we're given what the transmitted intensity is, 10 watts per square meter, and the incident intensity is one kilowatt per square meter. So, we'll divide both sides by I naught and then switch the sides around and we have cos squared theta equals I over I naught and then take the square root of both sides and so we have Cos theta equals the square root of the ratios of the intensities and then we'll take the inverse co sine of both sides to get the theta the inverse cosine of the square root of the ratio of intensities. So, it’s the inverse cosine of the square root of 10 watts per square meter divided by 1000 watts per square meter, the units here need to be the same by the way and to convert kilowatts into Watts here for the denominator and we end up with 84.3 degrees.
