Question
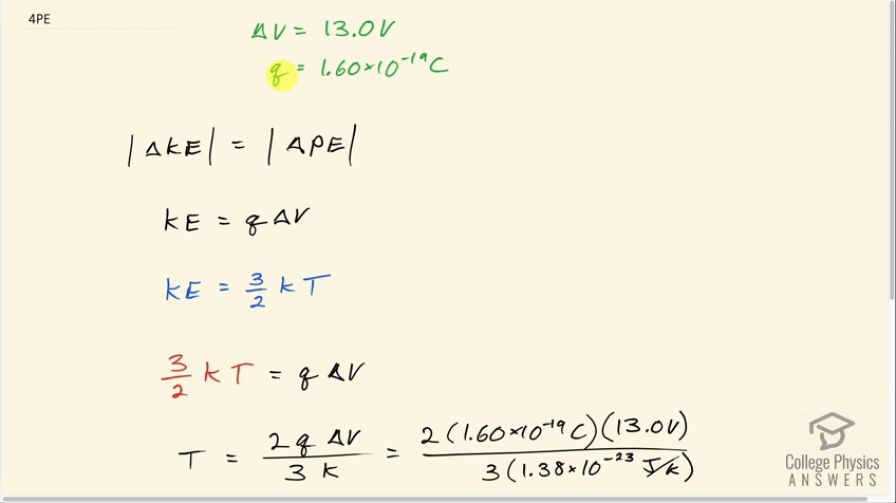
Singly charged gas ions are accelerated from rest through a voltage of 13.0 V. At what temperature will the average kinetic energy of gas molecules be the same as that given these ions?
Final Answer
Solution video
OpenStax College Physics, Chapter 19, Problem 4 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Some ions are singularly charged which is to say that each has an elementary charge on it so the charge of each ion is 1.60 times 10 to the minus 19 coulombs and they are accelerated through a voltage of 13.0 volts. So the amount of change in kinetic energy is equal to the amount of change in potential energy. I'm just putting these absolute value bars on here so that we can just ignore negative signs and say that the amount of kinetic energy that the thing gains equals the amount of potential energy that it lost and the amount of potential energy change is the charge times the change in voltage and this kinetic energy, we are asked at what temperature would these ions have the same kinetic energy? And so this is an expression from chapter 13 which says that kinetic energy due to thermal motion is 3 over 2 times Boltzmann's constant times the absolute temperature in Kelvin. So we can substitute 3 over 2 over kT in place of kinetic energy here and we get 3 over 2 kT equals q times ΔV and then multiply both sides by 2 over k to solve for T. So the temperature then is 2 times the charge on each ion times the change in potential difference divided by 3 times Boltzmann's constant. So that's 2 times 1.60 times 10 to the minus 19 coulombs times 13.0 volts divided by 3 times 1.38 times 10 to the minus 23 joules per Kelvin and that is 1.00 times 10 to the 5 Kelvin.
