Question
Fusion probability is greatly enhanced when appropriate nuclei are brought close together, but mutual Coulomb repulsion must be overcome. This can be done using the kinetic energy of high-temperature gas ions or by accelerating the nuclei toward one another. (a) Calculate the potential energy of two singly charged nuclei separated by by finding the voltage of one at that distance and multiplying by the charge of the other. (b) At what temperature will atoms of a gas have an average kinetic energy equal to this needed electrical potential energy?
Final Answer
Solution video
OpenStax College Physics, Chapter 19, Problem 10 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
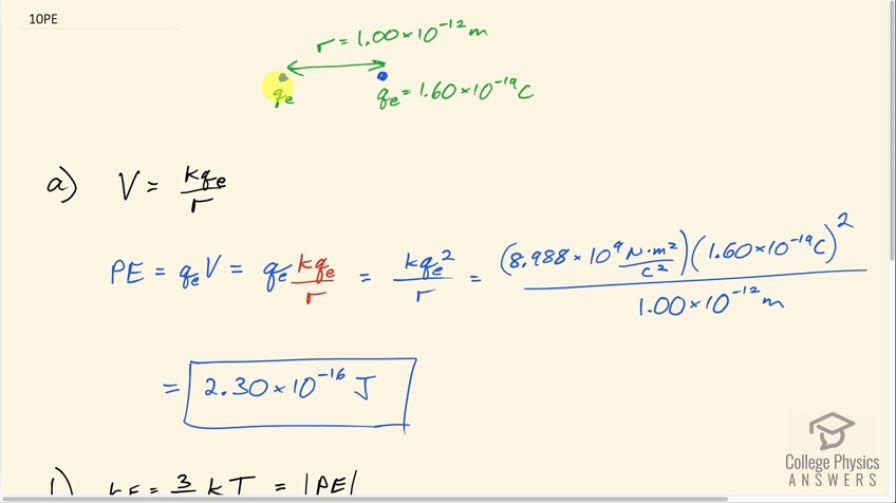
This is College Physics Answers with Shaun Dychko. So two singly charged nuclei need to be 1.00 picometer apart in order for fusion to be likely and the job in part (a) of this question is to figure out what is the potential energy of these two nuclei? So the potential at this position here, say due to the left charge, is the Coulomb's constant times this charge divided by the distance to that position and then the potential energy of a charge put here will be its charge multiplied by the potential due to the left charge. Now since the charges are the same— they are both the elementary charge, q e— we'll end up with q e squared here. So we have the potential energy then is Coulomb's constant times each of the charges multiplied together divided by the distance between them. So that's 8.988 times 10 to the 9 newton meters squared per coulombs squared times 1.60 times 10 to the minus 19 coulombs squared divided by 1.00 times 10 to the minus 12 meters which is 2.30 times 10 to the minus 16 joules Now part (b) asks, you know, because temperature is one method for causing the nuclei to get this close together because with increasing temperature, comes increasing kinetic energy and with kinetic energy, you know, this particle here could start at some, you know, basically infinite distance away some place where the potential energy is basically zero and then it needs enough kinetic energy such that the kinetic energy will decrease, decrease, decrease to the point where it's zero finally by this position—1.00 picometer away from this other nucleus— and so we need kinetic energy of equal magnitude to this potential energy here in order for the kinetic energy due to thermal movement to cause the nuclei to get this close together. So from chapter 13, we learn that the kinetic energy of a thermal motion is 3 over 2 times Boltzmann's constant times temperature and little bit confusing because it's the same letter k for both of these but these are completely different constants; up here, it's Coulomb's constant and down here, it's Boltzmann's constant. So all this equals the potential energy we found in part (a) and we can solve for T by multiplying both sides by 2 over 3k and we have the temperature then is 2 times the potential energy divided by 3 times Boltzmann's constant. So that's 2 times 2.3009 times 10 to the minus 16 joules divided by 3 times 1.38 times 10 to the minus 23 joules per Kelvin and this gives a temperature of 1.11 times 10 to the 7 Kelvin and this shows you why it is difficult to do fusion because this is 11 million Kelvin, which is a super-high temperature that, you know, it's very difficult to find materials that can tolerate temperatures like that with which to build a fusion reactor.