Question
Find the capacitance of a parallel plate capacitor having plates of area that are separated by 0.100 mm of Teflon.
Final Answer
Solution video
OpenStax College Physics, Chapter 19, Problem 53 (Problems & Exercises)
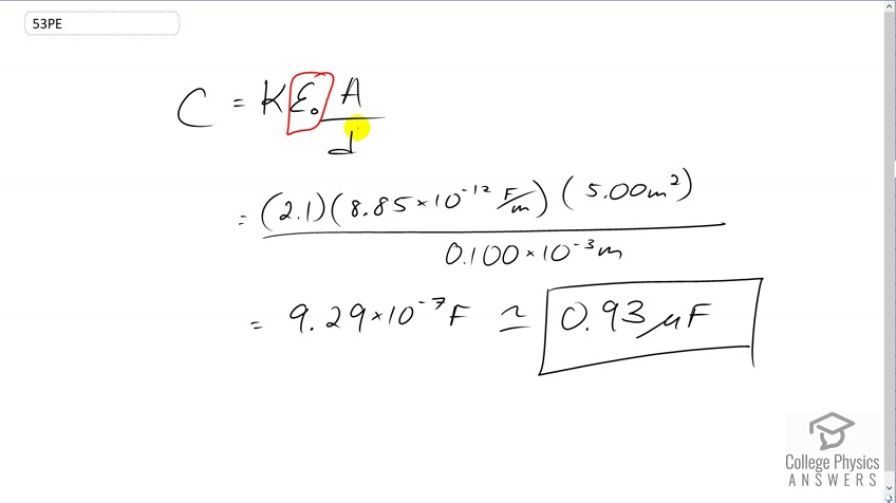
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The capacitance of a parallel plate capacitor is the permittivity of free space multiplied by the area of each plate divided by the separation between the plates. And since we have a dielectric material that is not a vacuum and not air, air is pretty much the same as a vacuum for dielectric, in this case we have teflon. So, we need to multiply by this Kappa, this dielectric constant. And so, the dielectric constant for teflon is 2.1, and we multiply that by permittivity of free space 8.85 times ten to the minus 12 farads per meter. And then, multiply by the area of five square meters and divided by 0.01 millimeters, which is 0.01 times ten to the minus three meters. And, this gives 0.93 microfarads as the capacitance.
Comments
Why do you use 2 sig figs in your final calculation?


