Question
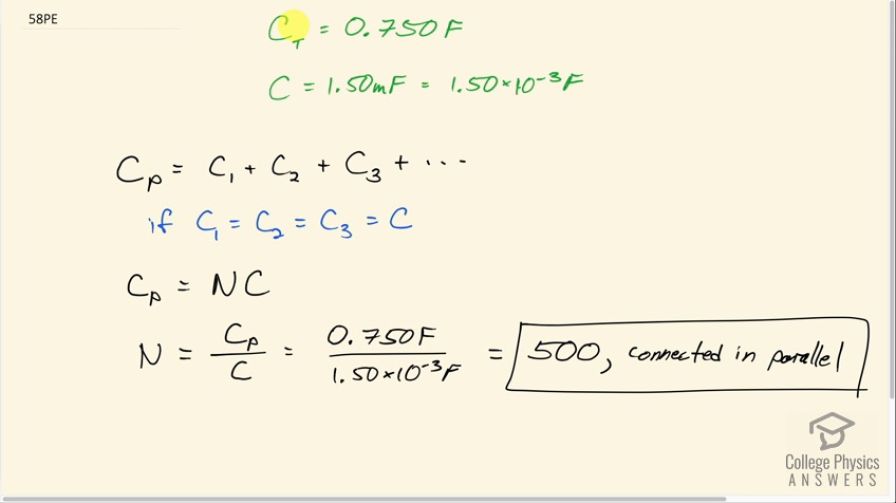
Suppose you want a capacitor bank with a total capacitance of 0.750 F and you possess numerous 1.50 mF capacitors. What is the smallest number you could hook together to achieve your goal, and how would you connect them?
Final Answer
500 capacitors connected in parallel.
Solution video
OpenStax College Physics, Chapter 19, Problem 58 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. Given as many little capacitors as we want, each with a capacitance of 1.50 millifarads, we want to create a total capacitance of 0.750 farads using as few as possible of these individual capacitors. Now when capacitors are connected in parallel, we get the greatest sum because we can just add the capacitances directly. So the first capacitor plus the second capacitor plus the third capacitor and so on makes the total capacitance when connected in parallel. Now all these capacitors are all the same, a whole bunch of these little ones of capacitance 1.50 millifarads and we will just call it C, in which case we have a whole lot of terms here—all letter C— in which case we have N of them and we can go N multiplied by C to get the total capacitance in parallel. So N is the number of capacitors that we are using and we will divide by the individual capacitance's and we get 0.750 farads divided by 1.50 times 10 to the minus 3 farads which is 500 capacitors, connected in parallel.