Question
Show that for a given dielectric material the maximum energy a parallel plate capacitor can store is directly proportional to the volume of dielectric ( ). Note that the applied voltage is limited by the dielectric strength.
Final Answer
Please see the solution video.
Solution video
OpenStax College Physics, Chapter 19, Problem 68 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Video Transcript
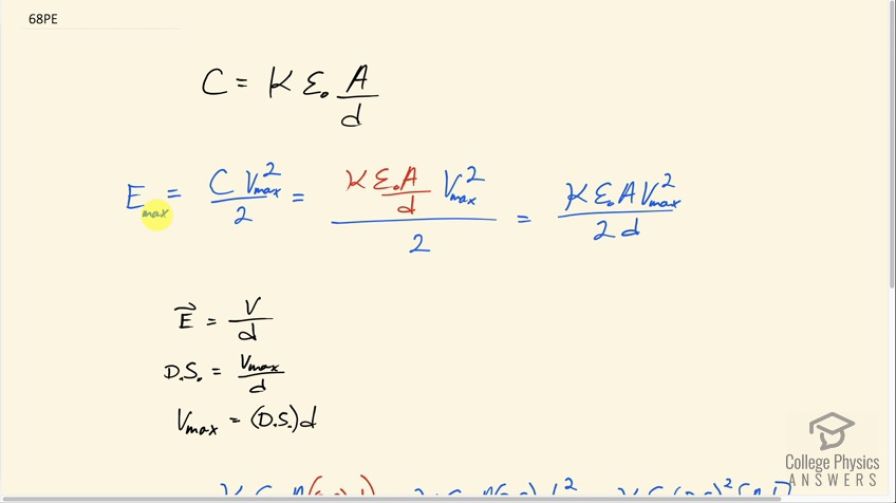
This is College Physics Answers with Shaun Dychko. We are going to show that the maximum amount of energy that can be stored in a capacitor is proportional to the volume of dielectric material between the plates of this parallel-plate capacitor. So the energy at its maximum is going to be the capacitance times the maximum voltage squared divided by 2 and the capacitance for a parallel-plate capacitor is this dielectric constant multiplied by the permittivity of free space times the area of the plates divided by the distance between them and so we can substitute that in place of C and then just make it look a little bit cleaner by making the denominator 2d here so we have Kε0AV max squared over 2d is the maximum energy. Now we have an area term here and in order to have a volume term in this expression, we need to have this V max in terms of distance in order to multiply... what we wanna do is multiply the area by the distance between the plates. So let's turn to this electric field formula between two parallel plates: it equals the voltage across the plates divided by the distance between them. Now at the maximum voltage, this electric gets a special name which is the dielectric strength and so the maximum voltage then is dielectric strength multiplied by the distance between the plates after you multiply both sides by d here so we can substitute this in place of maximum voltage and so this is squared by the way because that's what we have here and usually I write the stuff that's substituted in red and then the stuff that's leftover from the formula in blue. Okay! So the dielectric strength gets squared the distance between the plates gets squared because this exponent 2 applies to both the factors there and one of these distances divides by this distance in the denominator so we have d to the power of 1 in the numerator multiplied by this area and then multiplied by all this other stuff and this is what proportional means is that this energy maximum is some stuff multiplied by this other thing that we are interested in and this is volume. And so we have shown that the maximum energy stored in a capacitor is proportional to volume because we can write an expression for this maximum energy as some factors multiplied by the volume.