Question
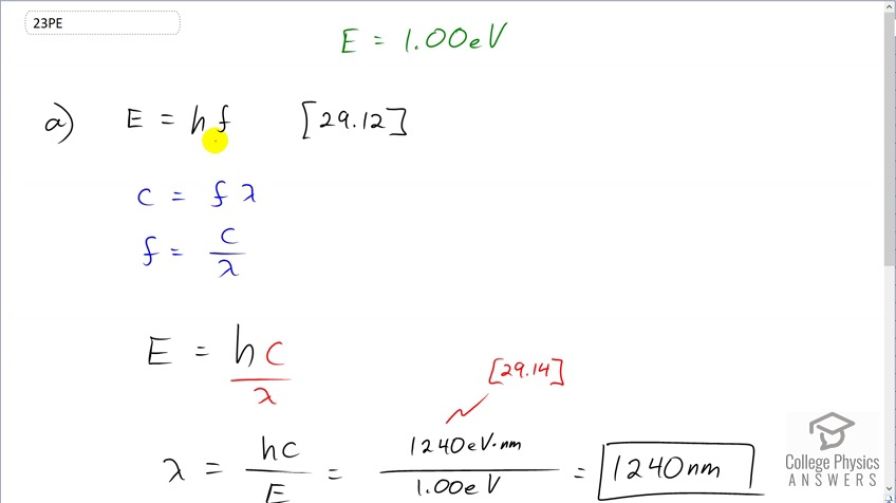
(a) What is the wavelength of a 1.00-eV photon? (b) Find its frequency in hertz. (c) Identify the type of EM radiation.
Final Answer
- 2.42 \times 10^{14} \textrm{ Hz}$
- This radiation is part of the infrared part of the spectrum. Red begins at .
Solution video
OpenStax College Physics, Chapter 29, Problem 23 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We want to know the wavelength of a 1 electron volt photon. So the energy of a photon is Planck's constant times its frequency; that's what equation [29.12] tells us. And then with the wave equation that says the speed of a wave equals its frequency times its wavelength, we can substitute for f. We'll divide both sides by the wavelength here and then switch the sides around and we get frequency is speed of light divided by its wavelength. And then we'll replace f with c over λ. So energy is Planck's constant times speed of light divided by λ and then we'll divide both sides by λ over energy to solve for λ. So the wavelength is Planck's constant times speed of light over the energy. So we choose 1240 electron volt nanometers as the units for this product hc, which is from [29.14] in the textbook. And we divide that by the 1 electron volt— energy that we are given— and these electron volts cancel, leaving us units of nanometers; that is 1240 nanometers. The frequency is going to be the energy divided by Planck's constant; that's what we can figure out here by dividing both sides by h here. We could have also used this formula and our answer for the wavelength to figure out the frequency, we could have gone speed of light divided by this, that would have been fine but I like to avoid using a number that I calculated in a subsequent calculation because maybe I made a mistake. So let's use numbers given to us in the question. So we have 1 electron volt divided by 4.14 times 10 to the minus 15 electron volt seconds giving us 1 over seconds, which is units of hertz, and that is 2.42 times 10 to the 14 hertz is the frequency. This radiation is part of the infrared part of the spectrum because it's a bit longer wavelength than the longest wavelength we can see, which is red about 750 nanometers.
