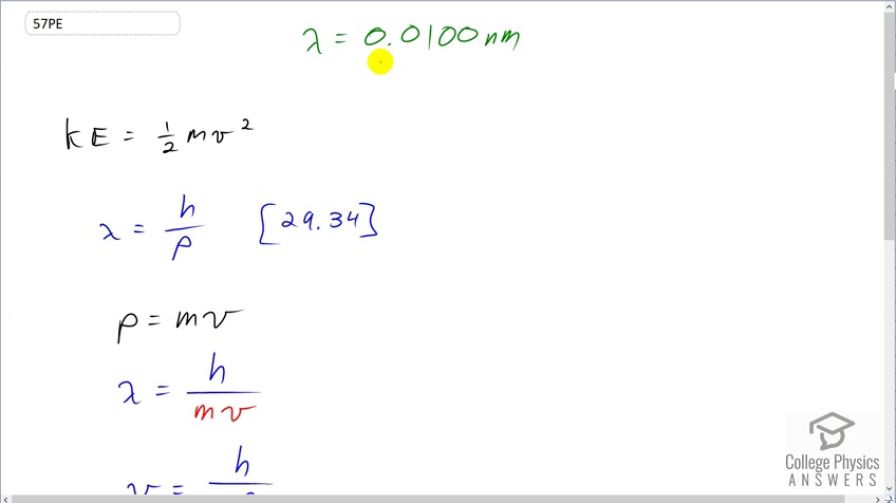
Question
What is the kinetic energy of an electron in a TEM having a 0.0100-nm wavelength?
Final Answer
Solution video
OpenStax College Physics, Chapter 29, Problem 57 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We want to know the kinetic energy of an electron in a tunneling electron microscope given that the electron has a de Broglie wavelength of 0.0100 nanometers. So kinetic energy is one-half mass times velocity squared and so we are going to need to substitute somehow for this velocity v. Well, we know the de Broglie wavelength of the electron is Planck's constant divided by momentum and momentum is mass times velocity. So we substitute mv in place of p here for the de Broglie wavelength and this can be rearranged to solve for v by multiplying both sides by v over λ. And so v is h over mλ and this can be substituted in for v in our kinetic energy formula. So we have kinetic energy of the electron is one-half times the mass times Planck's constant over mass times lambda squared and this works out to h squared over 2m λ squared. So here's Planck's constant squared divided by 2 times the mass of an electron times the wavelength of 0.01 nanometers, which is times 10 to the minus 9 meters, and we square that. And then this is gonna give us units of joules but we are told to find the energy in kiloelectron volts. So we multiply by 1 electron volt for every 1.602 times 10 to the minus 19 joules and then convert further by multiplying by 1 kiloelectron volt for every 10 to the 3 electron volts giving us 15.0 kiloelectron volts of kinetic energy for these electrons.
