Question
(a) How far away must you be from a 650-kHz radio station with power 50.0 kW for there to be only one photon per second per square meter? Assume no reflections or absorption, as if you were in deep outer space. (b) Discuss the implications for detecting intelligent life in other solar systems by detecting their radio broadcasts.
Final Answer
- 0.321 light years is not far in astronomical terms. Alien life would need to transmit at tremendous power in order for their radio transmissions to be detectable. Their radio signal also needs to be stronger than background noise in order to be distinguishable. Therefore it is unlikely that radio tansmissions from alien life will ever be detected.
Solution video
OpenStax College Physics, Chapter 29, Problem 35 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
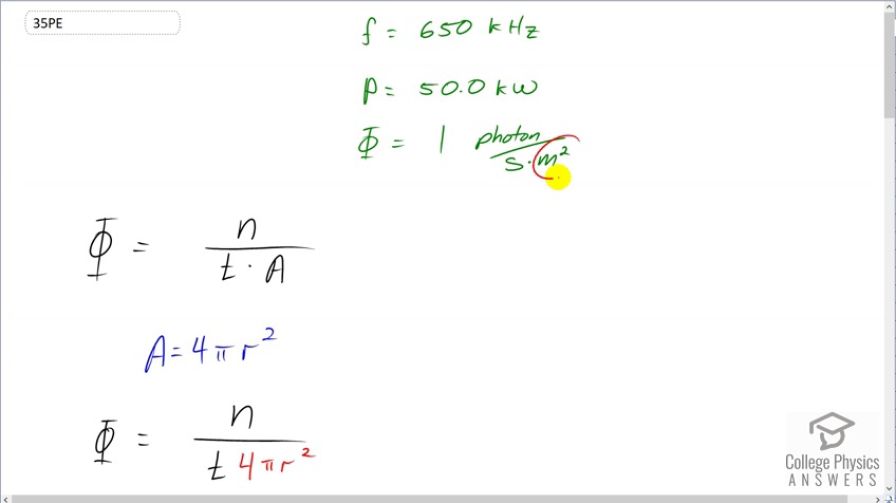
This is College Physics Answers with Shaun Dychko. We want to know how far from a radio station we need to be in order for only one photon to pass through a square meter every second. And this is a type of flux and this is the traditional symbol used for flux. Now, the transmission has a power of 50 kilowatts at a frequency of 650 kilohertz and this rate of transmission per area is going to be the number of photons per time per area and the area will be that of a sphere because this transmission tower is radiating uniformly in all directions and so the area at a distance r is gonna be area of a sphere which is 4πr squared and our question here is to figure out, what is r? And so the flux then is the number of photons per time times this area 4πr squared. Now, power is total energy transmitted per time and we are told what the power is— 50 kilowatts— and we can express this total energy as the number of photons multiplied by the energy per single photon and we have an expression for that, which is hf— Planck's constant times frequency. And this n over t then we can solve for it and say that it is power over Planck's constant times frequency by multiplying both sides by 1 over hf here. And then this is something we can substitute into our flux formula. So we are gonna replace n over t with P over hf. So the flux then is power over Planck's constant times frequency times 4πr squared and then we'll solve for r squared by multiplying both sides by r squared over flux. So r squared is P over 4πhfΦ and then we'll take the square root of both sides. So the distance from the transmission tower such that you have one photon per second through a square meter is the square root of 50 times 10 to the 3 watts over 4π times Planck's constant times the frequency—650 times 10 to the 3 hertz— times the flux which we are told is 1 photon per second per meter square. And that works out to this many meters and to put it into more astronomical units, we'll mutliply by 1 light-year for every 2.998 times 10 to the 8 meters per second times this many seconds in a year and that works out to 0.321 light years. So that's the distance from the radio station such that there will be one single photon every second through a square meter. And that is actually not very far in astronomical terms. And so alien life would need to transmit at very very high powers in order for the radio transmissions to be detectable because it'd be very difficult to deduct a single photon in a square meter because those photons have to compete with just the background photons that are just whizzing around in space anyway. It would be very difficult to separate that signal from the background noise and so it's very unlikely that radio transmissions from alien life will ever be detected.
