Question
The decay energy of a short-lived nuclear excited state has an uncertainty of 2.0 eV due to its short lifetime. What is the smallest lifetime it can have?
Final Answer
Solution video
OpenStax College Physics, Chapter 29, Problem 69 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
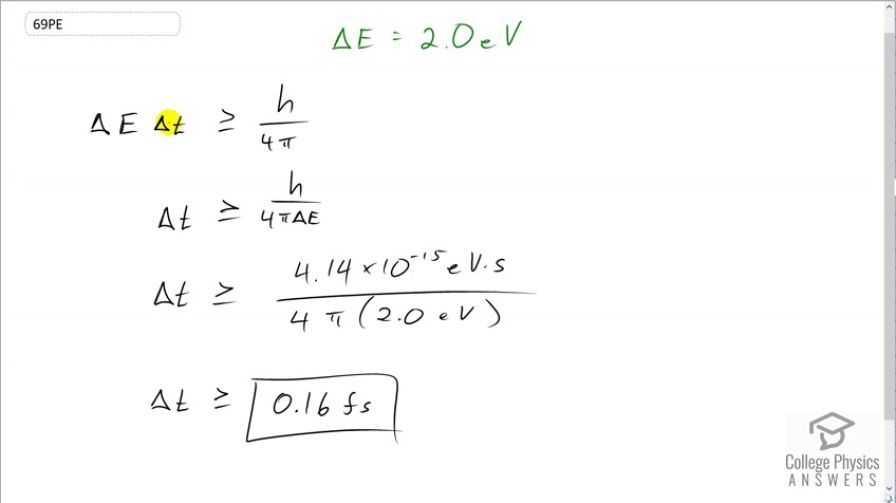
This is College Physics Answers with Shaun Dychko. The uncertainty in the decay energy of some nuclear state is 2 electron volts. And so that uncertainty multiplied by the uncertainty in time has to be greater than or equal to Planck's constant over 4π; this is the Heisenberg uncertainty principle. And that means that Δt then after we multiply both sides by 1 over ΔE here, is Planck's constant over 4π times ΔE. So the minimum uncertainty in time is 4.14 times 10 to the minus 15 electron volt seconds divided by 4π times 2 electron volts and this works out to 0.16 femtoseconds, is the minimum uncertainty in the time of this decay.
