Question
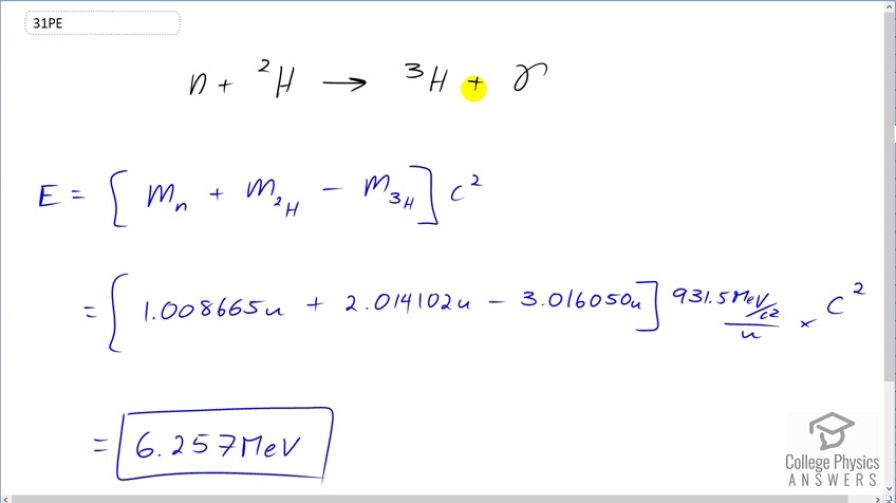
Tritium is naturally rare, but can be produced by the reaction . How much energy in MeV is released in this neutron capture?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 32, Problem 31 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Let's calculate the energy released in neutron capture by deuterium. So deuterium creates tritium and a gamma ray. Now to find the energy released, we find the difference in mass between what we start with and what we end with multiply by c squared; the gamma ray has no mass. So we have mass of the neutron plus mass of deuterium minus mass of tritium times c squared. Now these are atomic masses which include the mass of the associated electrons but that doesn't matter because there's one electron surrounding the deuterium atom and there's also one electron surrounding the tritium atom and so it's being subtracted away. So it's included here but then it's being subtracted away here and so there's nothing to worry about with the electrons here and the neutron of course has no electrons around it. So this is the mass of a neutron plus atomic mass of deuterium minus the atomic mass of tritium converted into megaelectron volts per c squared for every atomic mass unit and then we multiply by c squared and we end up with 6.257 megaelectron volts of energy released.
