Question
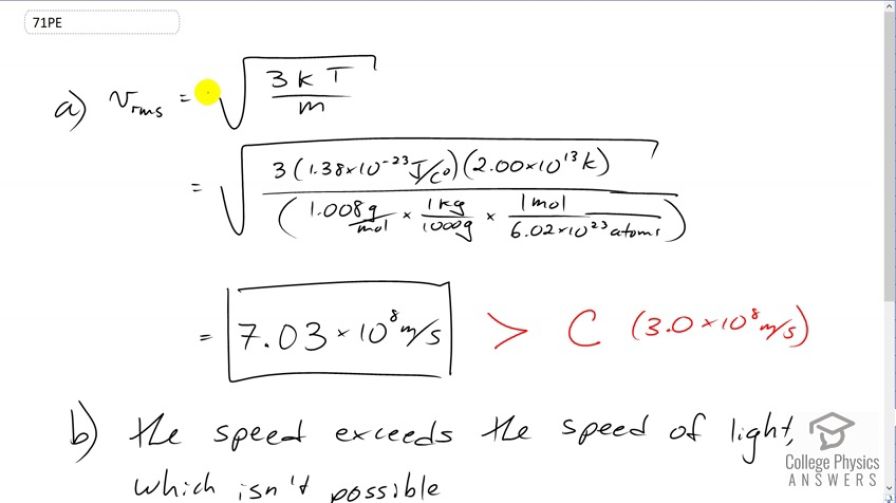
The temperature inside a supernova explosion is said to be . (a) What would the average velocity of hydrogen atoms be? (b) What is unreasonable about this velocity? (c) Which premise or assumption is responsible?
Final Answer
- The speed exceeds the speed of light. This is not possible.
- The ideal gas law doesn't apply at such high temperatures and pressures.
Solution video
OpenStax College Physics, Chapter 13, Problem 71 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
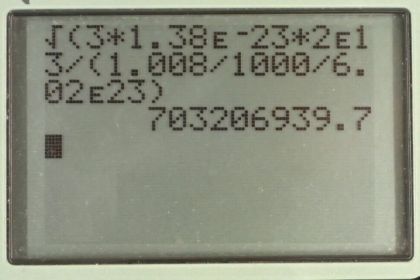
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We're going to find the root mean square velocity of hydrogen atoms in a supernova. So it's going to be the square root of three times Boltzmann's constant times the absolute temperature divided by the mass of the hydrogen atom. So that's square root of three times 1.38 times ten to the minus twenty-three joules per Celsius degree, times two times ten to the thirteen Kelvin which is what we're told the temperature in a supernova is, divided by the molar mass of hydrogen, 1.008 grams per mole which we convert into kilograms by multiplying by one kilogram for every 1000 grams. That gives us kilograms per mole and then we multiply by one mole for every 6.02 times ten to the twenty-three atoms, this is Avogadro's number, and the mole cancels and we're left with kilograms per atom. So one atom would have this many kilograms mass. All this works out to 7.03 times ten to the eight meters per second is the rms velocity. But that exceeds the speed of light which Einstein has shown is not possible. So that's what makes that unreasonable. The speed of light is three times ten to the eight meters per second. What we learn from this is that the ideal gas law does not apply at such high temperature and pressures.