Question
Large helium-filled balloons are used to lift scientific equipment to high altitudes. (a) What is the pressure inside such a balloon if it starts out at sea level with a temperature of and rises to an altitude where its volume is twenty times the original volume and its temperature is ?
(b) What is the gauge pressure? (Assume atmospheric pressure is constant.)
Final Answer
Solution video
OpenStax College Physics, Chapter 13, Problem 25 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
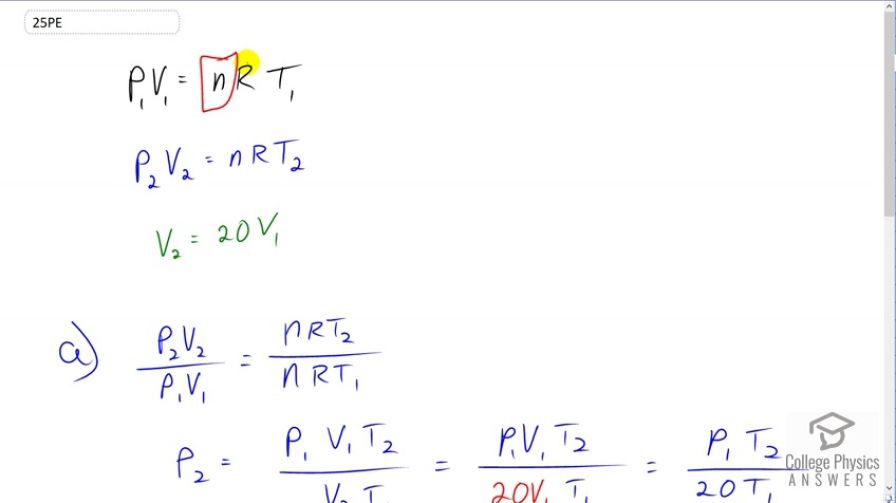
This is College Physics Answers with Shaun Dychko. When this Helium balloon is at sea level, it's pressure one times volume one will equal the number of moles of Helium gas in it times the gas constant times the temperature at sea level T1. And then when it's way up high in the atmosphere, it will have a different pressure different volume and a different temperature and I write a subscript two to label each of those. But the number of moles of Helium inside will be the same because it's a closed balloon and the gas constant is of course the same. So this does not get a subscript two. And we're told that volume two is 20 times volume one. So we're going to figure out what pressure two is by going P2V2 divided by P1V1, we're dividing the left sides of this equation. And that's going to equal the right sides of the equations divided, as well. So NRT2 over NRT1. And the NR is cancel because they're common factors. And then we'll solve for P2 by multiplying both sides by P1V1 over V2 and that gives us this line here. We have P2 is P1V1T2 over V2T1. Now, V2 we're told is 20 times V1 so we'll substitute for that. And now the V1 is cancel and we have pressure two way up high in the atmosphere equals pressure one at sea level times the temperature in the upper atmosphere divided by 20 times the temperature at sea level. And then we plug in numbers. We have to make an assumption about what this pressure must be. It doesn't explicitly tell us what the pressure is at sea level but we'll have to assume that it's one atmosphere. And in brackets, the question also says assume atmospheric pressure is constant which means that when the volume increases to 20 times in the upper atmosphere, that volume increase is part of this PV equals NRT Ideal Gas Law. And is not increasing in volume as result to the surrounding atmospheric pressure decreasing. So, the change in volume is only due to the Ideal Gas Law. So, at sea level, we'll assume the balloon is inflated to a pressure of one atmosphere and then we'll plug in the temperature is but convert them into Kelvin. So that's negative 50.0 degrees Celsius in upper atmosphere plus 273.15 to convert this into Kelvin. And then divided by 20 times ten degrees Celsius at sea level plus 273.15 and that gives 0.0394 atmospheres is the absolute pressure in the upper atmosphere inside the balloon. Then, the gauge pressure up there is going to be the absolute pressure that we calculated minus atmospheric pressure. So that's 0.0394 atmospheres minus one atmosphere which is negative 0.961 atmospheres.
