Question
A deep-sea diver should breathe a gas mixture that has the same oxygen partial pressure as at sea level, where dry air contains 20.9% oxygen and has a total pressure of . (a) What is the partial pressure of oxygen at sea level? (b) If the diver breathes a gas mixture at a pressure of , what percent oxygen should it be to have the same oxygen partial pressure as at sea level?
Final Answer
Solution video
OpenStax College Physics, Chapter 13, Problem 57 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
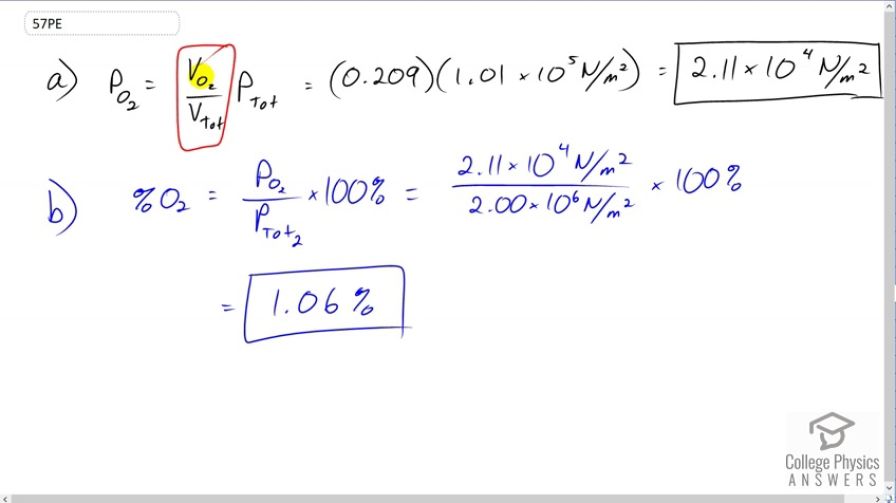
This is College Physics Answers with Shaun Dychko. The partial pressure of a gas in a mixture of gases is the fraction of the volume that that gas takes of the total multiplied by the total pressure. So, in regular atmosphere at atmospheric pressure, we have the percentage of the volume which is oxygen is 20.9 percent which as a decimal is 0.209. So we multiply that by atmospheric pressure to get 2.11 times ten to the four newtons per square meter is the partial pressure of Oxygen in the atmosphere. Now, in the scuba tank for this deep sea diver, we want the partial pressure of Oxygen to be the same as it is in the regular atmosphere. Now the pressure in the tank though is going to be higher in order to squish the air out into the hose and into the regulator when it's surrounded by this high pressure water under the sea. Now, so this total pressure is going to be different that it was before and so I put a subscript two on it to say that this is not the same as the total pressure in this equation here. And if we rearrange this to solve for the percent of the gas, and so I divided both sides by P total to get the percent of the volume percentage. So, the volume percentage of the Oxygen is going to be the partial pressure of Oxygen divided by the total pressure of the air in this tank. And that's 2.11 times ten to the four newtons per square meter we calculated in part a ivided by two times ten to the six newtons square meter. And that gives 1.06 percent of the volume should be Oxygen in this particular tank in order to have partial pressure of Oxygen as the diver used to at sea level.
