Question
Some incandescent light bulbs are filled with argon gas. What is for argon atoms near the filament, assuming their temperature is 2500 K?
Final Answer
Solution video
OpenStax College Physics, Chapter 13, Problem 39 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
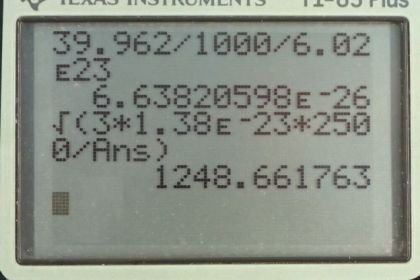
This is College Physics Answers with Shaun Dychko. The root mean squared velocity for an atom or molecule is the square root of three times Boltzmann's constant times the temperature in Kelvin, divided by the mass of one atom or molecule. So the mass of an argon atom is the molar mass that we looked up in Appendix A at the end of the textbook, 39.962 grams per mole. Then we have to convert this into kilograms per atom because we need to have MKS units in our formulas, so meters, kilograms, and seconds. So we multiply this by one kilogram for every 1000 grams and then multiply by one mole for every 6.02 times ten to the twenty-three atoms. This is Avogadro's number. This works out to 6.6382 times ten to the minus twenty-six kilograms per atom. So then we substitute numbers into our v rms formula. So the rms velocity of this argon atoms near the filament in this incandescent light bulb will be square root of three times 1.38 times ten to the minus twenty-three joules per Kelvin, times 2500 Kelvin divided by 6.6382 times ten to the minus twenty-six kilograms per atom giving a speed of 1.25 times ten to the three meters per second.