Question
An airplane passenger has of air in his stomach just before the plane takes off from a sea-level airport. What volume will the air have at cruising altitude if cabin pressure drops to ?
Final Answer
Solution video
OpenStax College Physics, Chapter 13, Problem 29 (Problems & Exercises)
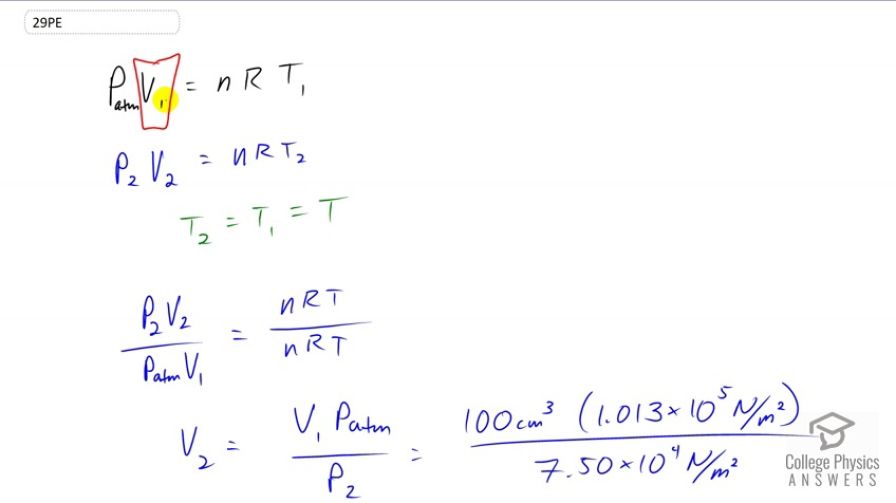
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. At sea level this passenger on the plane will be experiencing atmospheric pressure and will have some initial volume of air in their stomach of 100 cubic centimeters and call that V1. And that equals the number of moles of air times the gas constant times the temperature at sea level. And when the plane goes up to cruising altitude, it will have a different pressure P2 that we're given and we'll need to find out what is this volume two in their stomach. And that equals the same number of moles of gas in their stomach times the same gas constant times temperature two but I think we can make an assumption that the cabin of the plane will be designed to keep the same temperature for the comfort of the passengers. So we'll say that T2 and T1 are the same. And we'll write it as just T with no subscripts on this line here. So we're going to divide the two equations here, the blue and the black one. And we have P2V2 over P atmosphere V1 equals NRT2 but I just wrote T with no subscript there since T2 is the same as T1, divided by NRT1 but just T here. And this makes the number one. And we can solve for V2 by multiplying both sides by P atmosphere V1 over P2 and we're multiplying this one by that, as well. So V2 is V1 times atmospheric pressure divided by the pressure at cruising altitude. That's 100 cubic centimeters times 1.013 times ten to the five newtons per square meter divided by 7.5 times ten to the four newtons per square meter giving a volume of 135 cubic centimeters at cruising altitude.
Comments
How did you find Patm?
Hi Dposty123,
Thank you for the question. is a standard number that is defined. Here's the wikipedia entry: https://en.wikipedia.org/wiki/Atmospheric_pressure, and it's also mentioned in the textbook.


