Question
A bicycle tire has a pressure of at a temperature of and contains of gas. What will its pressure be if you let out an amount of air that has a volume of at atmospheric pressure? Assume tire temperature and volume remain constant.
Final Answer
Solution video
OpenStax College Physics, Chapter 13, Problem 33 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
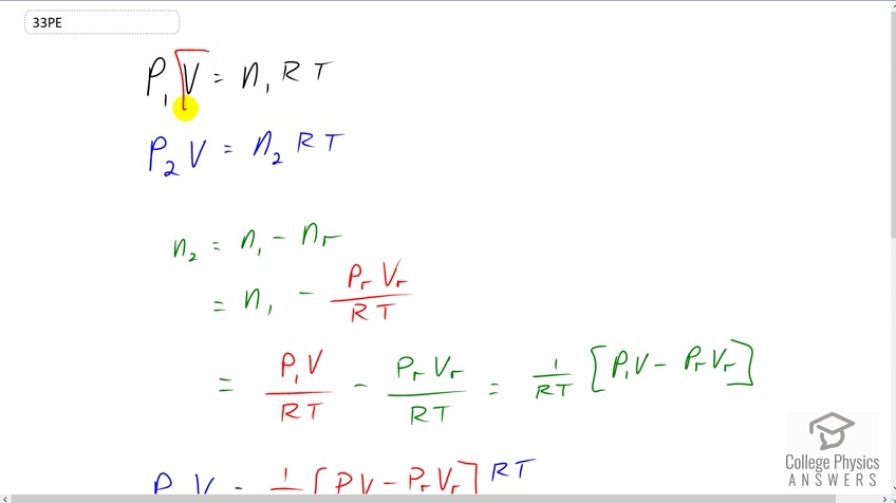
This is College Physics Answers with Shaun Dychko. Initially this bicycle tire has a pressure that we're given, we'll call it P one, and some volume, and the volume does not have a subscript because we're told to say that the volume is constant even when some air is let out of the tire, the volume of the tire does not change. So this P one V equals the initial number of moles of air particles in the tire, times the gas constant, times the temperature. The temperature we're told is also constant and so T does not have a subscript. Then after some gas is released, there is a new number of moles n two inside the tire, and then there's going to be a new pressure, we'll call it P two. So our job is to solve for P two. So we need to figure out what n two is. It's going to be the number of moles that were initially inside the tire, minus the number of moles removed. I have a subscript r for removed. The number of moles removed is going to be if you solve this for n, you can divide both sides by r T and you get the pressure of the gas removed, times the volume of the gas removed, and both of these things are given, and divided by r T, T being the temperature of the gas inside the tire. So the pressure of the gas removed is atmospheric pressure and the volume that's removed we're told is 100 cubic centimeters. Then we can also replace n one by rearranging this and we'll say n one equals P one V over r T by dividing both sides by r T. We write that substitution here. So n one is P one V over r T. Then we have a common factor of one over r T multiplied by P one V minus P removed volume removed. This we can plug in for n two in this formula. So I do that on this line. So P two V equals one over r T times P one V minus P r V r, all times r T. These r T's cancel and then we can divide both sides by V. So divide each term by V. So P two then is P one, cause the V's cancel there, minus the pressure of the gas removed, times the volume of gas removed divided by the volume of gas in the tire. So we have seven times ten to the five newtons per square meter initial pressure, minus atmospheric pressure times 100 cubic centimeters converted into cubic meters by multiplying by one meter for every 100 centimeters three times. Then divide by two liters which we have to convert into cubic meters in order to be consistent with these units here. So we multiply two liters by one cubic meter for every 1000 liters. We end up with 6.95 times ten to the five newtons per square meter is the pressure in the tire after 100 cubic centimeters of gas is removed.
