Question
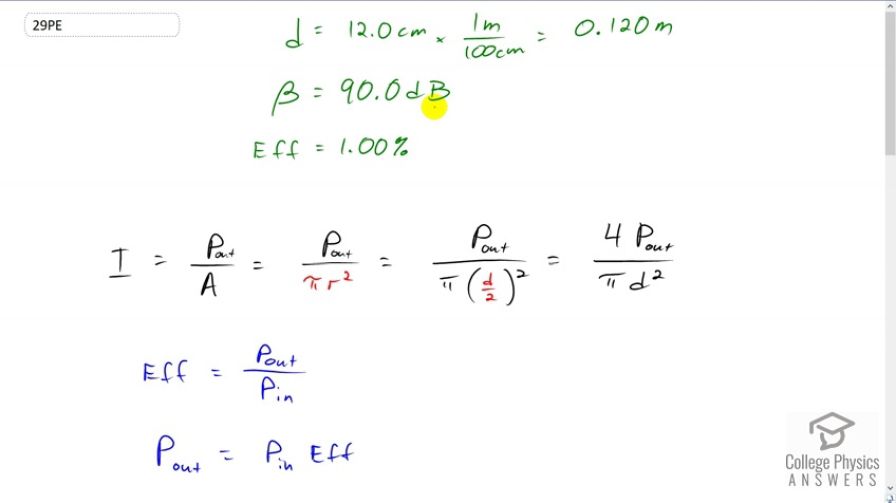
Loudspeakers can produce intense sounds with surprisingly small energy input in spite of their low efficiencies. Calculate the power input needed to produce a 90.0-dB sound intensity level for a 12.0-cm-diameter speaker that has an efficiency of 1.00%. (This value is the sound intensity level right at the speaker.)
Final Answer
Solution video
OpenStax College Physics, Chapter 17, Problem 29 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We are going to calculate the power input needed to create a sound level of 90 decibels output from a loud speaker which has an efficiency of only one percent given the diameter of the speaker cone is 12 centimeters. Lets begin by converting that into meters because our formula is going to need units of meters and that’s multiplied by one meter for every 100 centimeters, we get 0.120 meter. Now, intensity is the power output divided by the area and we are dealing with location directly in front of the loud speaker we told and the area is going to be the, the area of the loud speaker which was assumed to be in the shape of a circle. So, its pi times radius square, we’re given diameter, so we will substitute d over two in place of r squared or should say d over two in place of r and then that fraction become squared and the factor four appears in the numerator here, we have four times power output divided by pi times diameter squared of the loud speaker. Now, we want to know the power input and so lets replace this power output with an expression containing P in. Now, we know the efficiency is power output divided by power input and we will solve this for P out by multiplying both sides by P in and we have P out is P in times efficiency and so replacing P out with this, we get intensity as four times P in times efficiency divided by pi d squared. Now, we want to know what the P in is and so lets solve for it by multiplying both sides by pi d squared over four times efficiency on both sides and switch the sides around as well and we get power input is pi d squared I over four times efficiency. Now , we don’t know what the intensity is but we can... We can substitute for it though in terms of sound level because we do know the sound level is 90 decibels and so lets rearrange our decibels formula to solve for I so we are gonna divide both sides by ten and so we have logarithm of intensity divided by reference intensity is the sound level divided by ten and then make both sides exponent of ten and so we have I over I naught equal sound level to the power of sound level over ten and then multiply both sides by the reference intensity and you get an expression for intensity in terms of sound level. So, this is what we substitute in place of I in our P in formula. So, I have written that substitution in red here and now we know everything in this formula and we can solve for P in. So, its pi times 0.120 meters diameter squared times reference intensity which is ten to the minus twelve watt per square meter times ten to the power of 90 over 10, all divided by four times the efficiency of one percent which is 0.0100 and that gives a power input of only 1.13 times ten to the minus three watts.
