Question
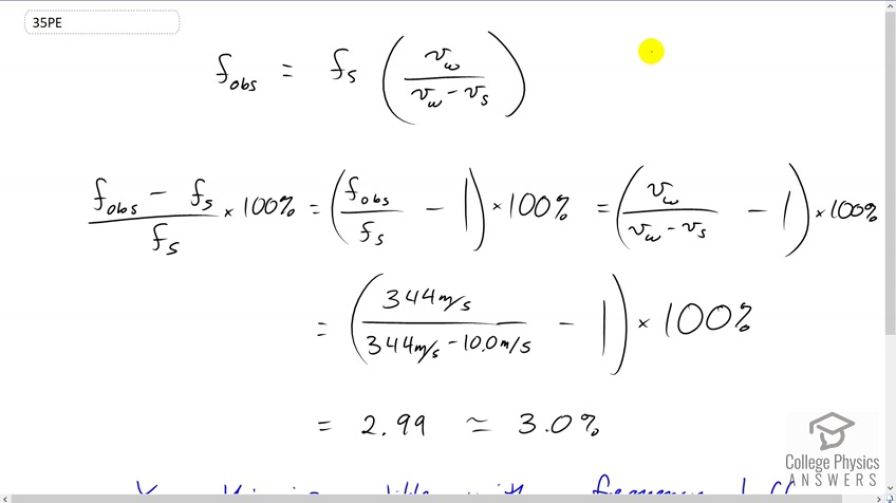
Can you perceive the shift in frequency produced when you pull a tuning fork toward you at 10.0 m/s on a day when the speed of sound is 344 m/s? To answer this question, calculate the factor by which the frequency shifts and see if it is greater than 0.300%.
Final Answer
Yes, this frequency difference is audible since it is 10 times the minimum difference of 0.30%.
Solution video
OpenStax College Physics, Chapter 17, Problem 35 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. When pulling a tuning fork towards you at ten meters per second, we want to see whether or not the doppler shift is going to be observable. So let's find the percent change in frequency and see if that's more than the minimal detectable. And so if the frequency changes by 0.3 percent or more, we will be able to detect it. It turns out, it's going to be changing by three percent. So, the doppler shifted frequency that you'll observe is the source frequency of the tuning fork multiplied by the speed of sound divided by this wave's speed of sound minus the speed of the source. And we have a minus here because when a source is moving towards the observer, it causes an increase in the observed frequency, and that means we need to have a minus here in order to make the denominator of this fraction smaller so that the whole fraction becomes bigger. So, we're going to have the difference in frequencies divided by the source frequency multiplied by 100 percent to get the percent change frequency. And then, substitute, well first, divide each factor by this denominator, so that's F observer divided by F source minus F source divided by F source, which is one. And then, replace F observed divided by Fs with this, since if you were to divide this by Fs the Fs will cancel, and you'll be left with this fraction. So, we have speed of sound divided by the speed of sound minus the speed of the tuning for minus one times 100 percent. So, that's 344 meters per second divided by 334 minus ten meters per second minus one times 100 percent, which works out to about 3.0 percent. And that is ten times the minimum audible difference of 0.30 percent, and so yes, this doppler shift is detectable and audible.
