Question
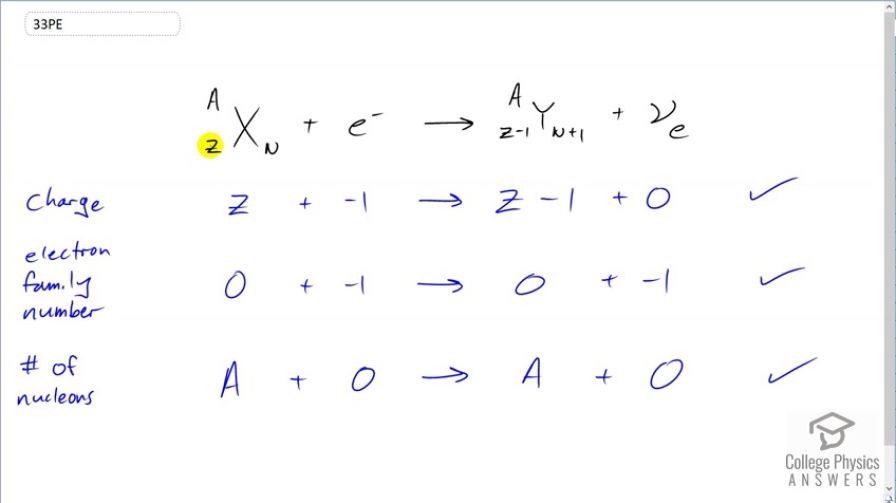
Confirm that charge, electron family number, and the total number of nucleons are all conserved by the rule for electron capture given in the equation . To do this, identify the values of each before and after the capture.
Final Answer
Please see the solution video.
Solution video
OpenStax College Physics, Chapter 31, Problem 33 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. During electron capture, an electron enters the nucleus and combines with a proton to turn it into a neutron. So a proton is going to be lost and so this daughter nuclide has 1 less proton; the atomic number has been reduced by 1 in other words and it has 1 extra neutron. Now we are gonna check to make sure charge, electron family number and number of nucleons are conserved which means we'll find the total of each on both sides of this equation. So consider charge; we have positive charge in amount Z the atomic number and then we have a charge negative 1 from this electron so this is a total of Z minus 1. Now on the right hand side, we have no charge in the electron-neutrino and the daughter nuclide has a charge of Z minus 1. So Z minus 1 on both sides and that's why it checks out. Then looking at electron family number, this is not an electron and so it's electron family number is 0. The electron has an electron family number of negative 1 and then on the right hand side, we have 0 and negative 1 is the electron family number of an electron-neutrino. And that's actually the explanation for why this neutrino is created in order to conserve electron family number. And then the number of nucleons is A on the left side and of course the number is 0 for an electron that's not a nucleon and then there are A nucleons in the daughter nuclide as well. And there we go; that checks out as well.