Question
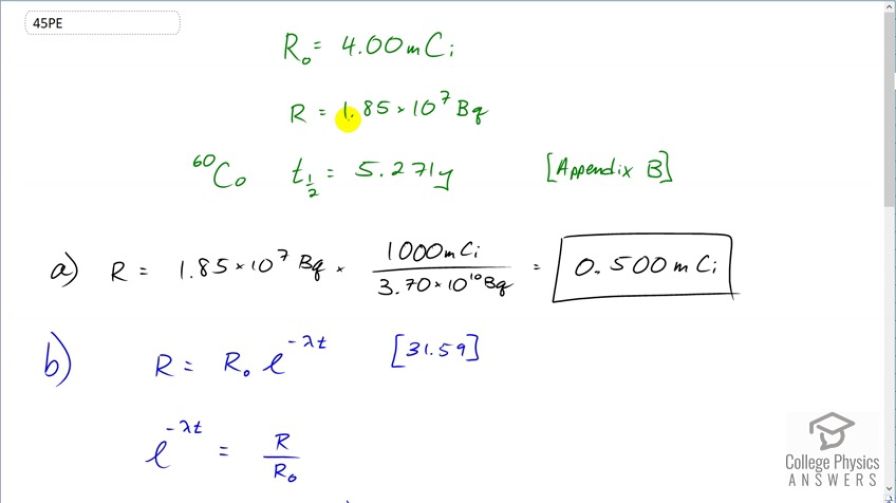
A source is labeled 4.00 mCi, but its present activity is found to be . (a) What is the present
activity in mCi? (b) How long ago did it actually have a 4.00-mCi activity?
Final Answer
Solution video
OpenStax College Physics, Chapter 31, Problem 45 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
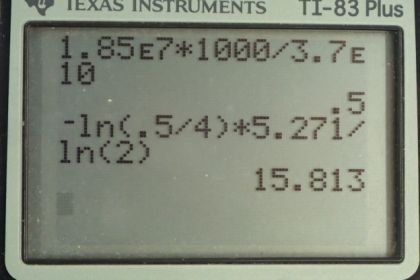
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A cobalt-60 sample is labeled with an activity of 4 millicuries but in actual fact, it has an activity of 1.85 times 10 to the 7 becquerels. Although until we convert this becquerels into units of millicuries, we can't really compare them but we are gonna find in part (a) that the activity is actually 0.500 millicuries and so the sample is pretty old; some time has passed such that the activity has reduced from the initial 4 millicuries to the present 0.500 millicuries. The half-life of cobalt-60 is 5.271 years and we'll use this equation in part (b) to figure out how old the sample is; at what time did it have this initial activity of 4 millicuries. So we know the activity is the initial activity times e to the negative decay constant times time— this is equation 59 from chapter 31— and we'll divide both sides by R naught and switch the sides around. And we get e to the negative λt equals R over R naught and then we'll take the natural logarithm of both sides. And the natural logarithm of e to the power of something is the something, the exponent. So we have negative λt on the left and on the right side, we have natural logarithm of R over R naught and then we'll divide both sides by negative λ. And so we have the time is negative ln— you might say ln instead of natural logarithm— negative ln of R over R naught all over λ and then we can replace this λ with natural logarithm of 2 divided by half-life; this is equation 37. Now since we are dividing by this λ or in other words dividing by this fraction, we'll instead multiply by its reciprocal in order to avoid dividing a fraction by a fraction because that gets messy so we are multiplying this by the reciprocal of this λ so that's what we have here. So we have log of R over R naught times the half-life divided by log 2 negative. So the time at which the sample had its initial activity was the negative log of 0.500 millicuries— present activity— divided by 4 millicuries—initial activity— times 5.271 years divided by log of 2 and we can use non-mks units here because it does work out in the end; these millicuries cancel. Normally becquerels is the unit that you would use because that's the mks unit for activity it's becquerels but we can use millicuries here just because they cancel anyway; the important thing is when dividing these units have to be the same whatever they are. And then we'll have our units of years in our final answer and we get 15.8 years is how old the sample is.