Question
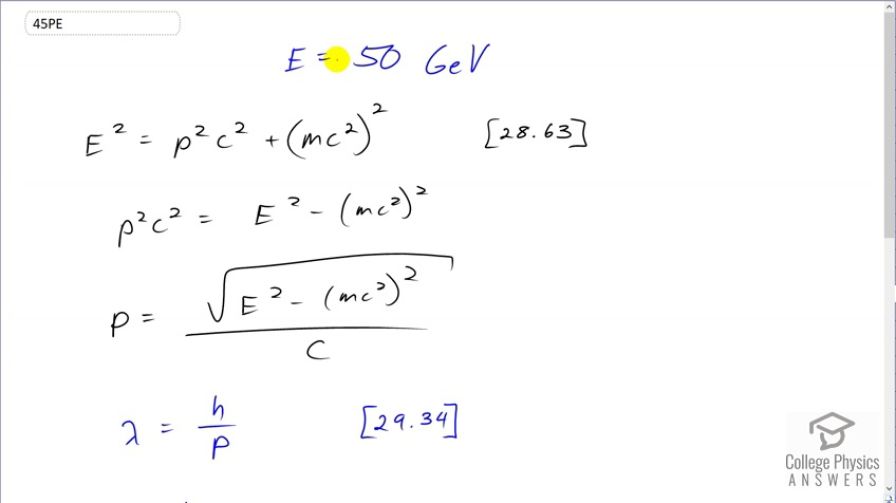
What is the wavelength of a 50-GeV electron, which is produced at SLAC? This provides an idea of the limit to the detail it can probe.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 33, Problem 45 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. At the Stanford Linear Accelerator, they create electrons with an energy of 50 gigaelectron volts and the question is what is the de Broglie wavelength of these electrons? So from chapter 29, equation 34 the wavelength is Planck's constant divided by the momentum of the particle and so the first thing we need to figure out is what is the momentum of these electrons. So in chapter 28, equation 63, we see that the total energy of an electron squared is the momentum of the electron squared times c squared plus its mass energy squared. And we'll solve this for p by subtracting mc squared squared from both sides and then dividing both sides by c squared and then also taking the square root of both sides. And so we have momentum then is the square root of the energy squared minus mc squared squared all divided by c. So this is what we substitute into the de Broglie wavelength equation; I guess normally I would do substitutions in red wouldn't I. So we are dividing by the wavelength which is the same as multiplying by the reciprocal of this fraction so I'm multiplying by the reciprocal of it so I'm writing the c on top then the square root of energy squared minus mass times c squared squared. So we substitute in Planck's constant and times the speed of light divided by square root of the energy written as 50 times 10 to the 9 electron volts converted into joules because we need mks units and then square that and then minus the energy of the electron converted also into joules and then square that and we end up with 2.5 times 10 to the minus 17 meters is the de Broglie wavelength of electrons in this accelerator.
