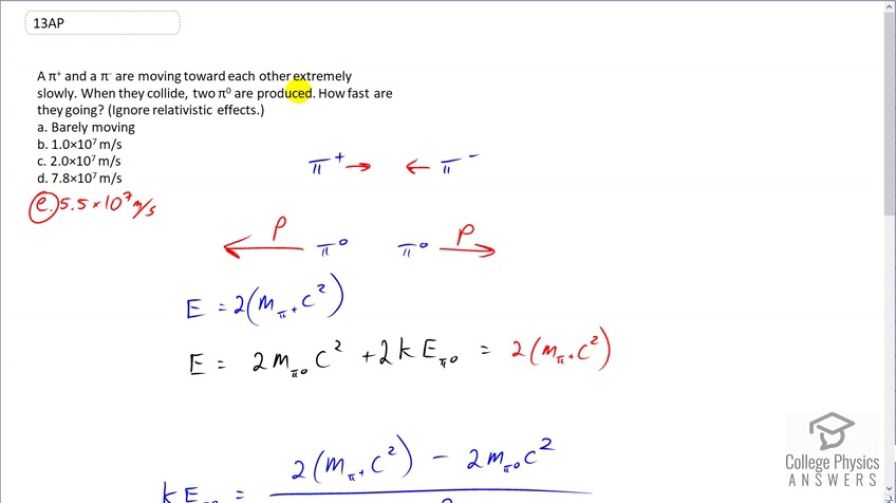
Question
A and a are moving toward each other extremely slowly. When they collide, two are produced. How fast are they going? (Ignore relativistic effects.)
- Barley moving
Final Answer
(e)
Solution video
OpenStax College Physics for AP® Courses, Chapter 33, Problem 13 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
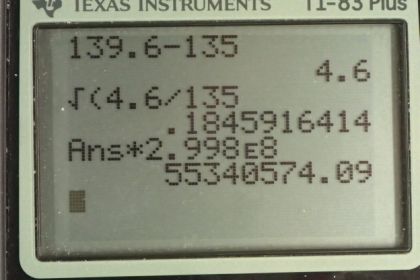
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A positive pion and a negative pion are approaching each other very slowly and what it's trying to get at when it says that is that assume that it has no kinetic energy and so the total energy of this system to begin with is just the rest mass energy of the positive and negative pions. After they collide, they will turn into two neutral pions and the question is how fast will these pions be going? Now they will be moving and they will be moving quickly because there is a difference in the total rest mass energy between these two systems. The total rest mass energy of the neutral pions is less than the rest mass energy of the positive and negative pions that we started with and so that difference has to get turned into a different form of energy which will be kinetic energy. Now these neutral pions have to have zero total momentum because we had zero total momentum to begin with essentially and so that means they are gonna be moving in opposite directions. Now since they have the same mass, they are gonna be moving at equal speeds because the momentum is mv in each case is gonna be m—mass of the neutral pion— times speed. Now what we are going to look at then is the total energy and then figure out the kinetic energy and then based on the kinetic energy of a neutral pion, we'll find its speed. OK So total energy is 2 times the mass of a positive pion times c squared because the positive and the negative pions have equals masses so we'll just take 2 times one of them. And the total energy after the collision is gonna be 2 times the rest mass energy of a neutral pion because there are two of them. Plus the kinetic energy of each neutral pion times by 2 since there are two particles moving. And this total has to equal the total energy that we started with. So we solve this for kinetic energy by taking this term to the right-hand side by subtracting it from both sides and then divide both sides by 2 and so one of the 2's cancel. So we have the mass of a positive pion— 139.6 megaelectron volts per c squared— times c squared minus the mass of a neutral pion— 135 megaelectron volts per c squared— multiplied by c squared and that difference is 4.6 megaelectron volts. So that's the kinetic energy of a single neutral pion and then we say that since we are told to ignore relativistic effects, we are meant to say that this kinetic energy equals mass of the neutral pion times its speed squared and so we solve this for v in order to finally answer our question; how fast are the neutral pions going? So we divide by the mass on both sides and then take the square root of both sides and we have the speed then is square root of the kinetic energy divided by the mass. So that's 4.6 megaelectron volts divided by 135 megaelectron volts per c squared square rooted and so 4.6 divided by 135 square rooted is 0.18459 and we are left with this factor being multiplied by c since we have, you know, c squared in the denominator of this denominator. Anyway so you can multiply top and bottom by c squared so these cancel there and we are left with c squared on the top and then square rooting that gives us the c. So this works out to 5.5 times 10 to the 7 meters per second so I have substituted the speed of light there multiplied it by this factor and turns out that that is not one of the options that the textbook gives us and so we write it in letter (e) 5.5 times 10 to the 7 meters per second and we can be sure that this is correct answer because you can take this speed and work backwards and come up with the correct total energy.