Question
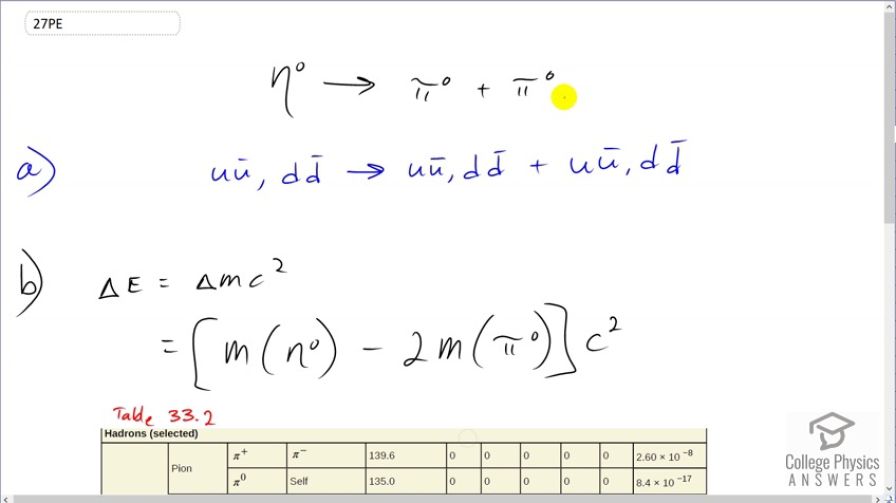
One decay mode for the eta-zero meson is
(a) Write the decay in terms of the quark constituents.
(b) How much energy is released?
(c) What is the ultimate release of energy, given the decay mode for the pi zero is ?
Final Answer
- Please see the solution video
Solution video
OpenStax College Physics for AP® Courses, Chapter 33, Problem 27 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The eta zero particle can turn into two neutral pions and to write this decay in terms of quark constituents, the eta zero is a mixture of up and anti-up as well as down and anti-down quarks and then that turns into these neutral pions which each are also a mixtures of the same pairs of quarks; up and anti-up or down and anti-down. And so you can think of an eta minus or sorry eta zero particle as being an excited state of the neutral pion because the quark constituents are the same. The change in energy in this decay is going to be the mass difference between the single eta zero particle and the combined mass of the two neutral pions. And so we have to look up these masses in table [33.2] and we see that the eta zero particle has a mass of 547.9 megaelectron volts per c squared and then each neutral pion has a mass of 135.0 megaelectron volts per c squared and that gives a difference of 277.9 megaelectron volts. But the total energy released in the decay of the eta zero particle is going to be more than this since those neutral pions will in turn decay to a pair of gamma rays each. So the total net decay then after enough time has passed is gonna be that the eta zero turns into four gamma rays. And so the mass difference in this decay is going to be just the mass of eta zero since gamma rays have no mass. And so the change in energy then where the energy released in this decay is gonna be the energy of the eta zero particle which is 547.9 megaelectron volts.
