Question
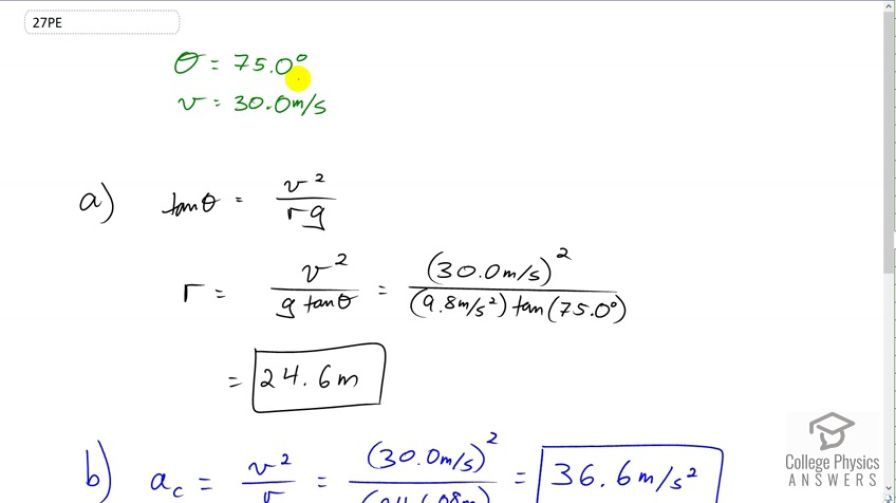
- What is the radius of a bobsled turn banked at 75.0° and taken at 30.0 m/s, assuming it is ideally banked?
- Calculate the centripetal acceleration.
- Does this acceleration seem large to you?
Final Answer
a)
b)
c) . This seems reasonable as it would not cause injury.
Solution video
OpenStax College Physics for AP® Courses, Chapter 6, Problem 27 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We begin by writing down the information we're given. We're told that the curve the bobsledder goes around is banked at an angle of 75 degrees and they proceed at 30 meters per second. The tangent of the ideal banking angle is equal to the speed squared divided by the radius of curvature, times the acceleration due to gravity. We can rearrange this to solve for r by multiplying both sides by r over tan theta. On the left the tangents cancel and on the right the r's cancel and we're left with r equals v squared over g tan theta. So that's 30 meters per second squared divided by 9.8 meters per second squared times tangent of 75. This gives 24.6 meters must be the radius of curvature. The centripetal acceleration that the bobsledder will experience is v squared over r, so that's 30 meters per second squared divided by this radius we just calculated, 24.608 meters. That's 36.6 meters per second squared. Part C asks us if this centripetal acceleration is reasonable and so to get some perspective on it, we'll divide it by a number that we're familiar with which is g, the acceleration due to gravity and see how many times this is compared to g. So 36.6 divided by 9.8 is 3.7. So, the acceleration that this bobsledder experiences is greater than acceleration due to gravity by a factor of 3.7. That seems reasonable because that should not cause injury.
