Question
(a) What is the period of rotation of Earth in seconds? (b) What is the angular velocity of Earth? (c) Given that Earth has a radius of at its equator, what is the linear velocity at Earth's surface?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 6, Problem 4 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
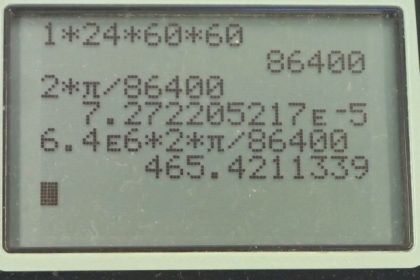
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The period of Earth's rotation is the number of seconds it takes for 1 day. So we multiply by 24 hours per day leaving us with units of hours times by 60 minutes per hour leaving us with units of minutes and then convert that into seconds by multiplying by 60 seconds per minute. So we are left with seconds as a unit and so we have 1 times 24 times 60 times 60 which is 86400 seconds per day. The angular speed of the Earth is the number of radians through which it rotates which is 2π radians— that's 1 full day of rotation— divided by the time it takes to do that which is 86400 seconds. So the angular speed then is 7.27 times 10 to the minus 5 radians per second. The linear speed on a point at the equator on the surface of the Earth is going to be the radius of the Earth multiplied by this angular speed. So that's 6.4 times 10 to the 6 meters—radius— times 2π radians over 86400 seconds which is 470 meters per second.